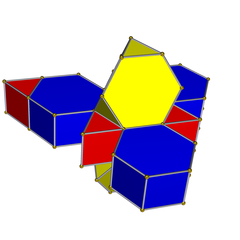
Truncated tetrahedral prism

This article needs additional citations for verification. Please help by adding citations to reliable sources. Unsourced material may be challenged and removed. Find sources:  –  ·  ·  · scholar · JSTOR (July 2014) (Learn how and when to remove this template message)

Truncated tetrahedral prism
Schlegel diagram
Type	Prismatic uniform polychoron
Uniform index	49
Schläfli symbol	t0,1{3,3}×{}
Coxeter-Dynkin
Cells	10: 2 3.6.6 4 3.4.4 4 4.4.6
Faces	24: 8 {3} + 18 {4} + 8 {6}
Edges	48
Vertices	24
Vertex figure	Isosceles-triangular pyramid
Symmetry group	[3,3,2], order 48
Properties	convex

In geometry, a truncated tetrahedral prism is a convex uniform polychoron (four-dimensional polytope). This polychoron has 10 polyhedral cells: 2 truncated tetrahedra connected by 4 triangular prisms and 4 hexagonal prisms. It has 24 faces: 8 triangular, 18 square, and 8 hexagons. It has 48 edges and 24 vertices.

It is one of 18 uniform polyhedral prisms created by using uniform prisms to connect pairs of parallel Platonic solids and Archimedean solids.

Net

Alternative names[]

1. Truncated-tetrahedral dyadic prism (Norman W. Johnson)
2. Tuttip (Jonathan Bowers: for truncated-tetrahedral prism)
3. Truncated tetrahedral hyperprism

External links[]

• 6. Convex uniform prismatic polychora - Model 49, George Olshevsky.
• Klitzing, Richard. "4D uniform polytopes (polychora) x x3x3o - tuttip".

This 4-polytope article is a stub. You can help Wikipedia by .

• v
• t