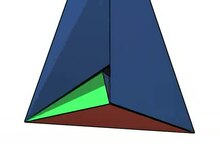
Császár polyhedron

Császár polyhedron
Play media An animation of the Császár polyhedron being rotated and unfolded
Type	Toroidal polyhedron
Faces	14 triangles
Edges	21
Vertices	7
Euler char.	0 (Genus 1)
Vertex configuration	3.3.3.3.3.3
Symmetry group	C1, [ ]+, (11)
Dual polyhedron	Szilassi polyhedron
Properties	Nonconvex

In geometry, the Császár polyhedron (Hungarian: [ˈt͡ʃaːsaːr]) is a nonconvex toroidal polyhedron with 14 triangular faces.

This polyhedron has no diagonals; every pair of vertices is connected by an edge. The seven vertices and 21 edges of the Császár polyhedron form an embedding of the complete graph ${\displaystyle K_{7}}$ onto a topological torus. Of the 35 possible triangles from vertices of the polyhedron, only 14 are faces.

Complete graph[]

The tetrahedron and the Császár polyhedron are the only two known polyhedra (having a manifold boundary) without any diagonals: every two vertices of the polygon are connected by an edge, so there is no line segment between two vertices that does not lie on the polyhedron boundary. That is, the vertices and edges of the Császár polyhedron form a complete graph.

If the boundary of a polyhedron with v vertices forms a surface with h holes, in such a way that every pair of vertices is connected by an edge, it follows by some manipulation of the Euler characteristic that

This equation is satisfied for the tetrahedron with h = 0 and v = 4, and for the Császár polyhedron with h = 1 and v = 7. The next possible solution, h = 6 and v = 12, would correspond to a polyhedron with 44 faces and 66 edges, but it is not realizable as a polyhedron. It is not known whether such a polyhedron exists with a higher genus (Ziegler 2008).

More generally, this equation can be satisfied only when v is congruent to 0, 3, 4, or 7 modulo 12 (Lutz 2001).

History and related polyhedra[]

The Császár polyhedron is named after Hungarian topologist Ákos Császár, who discovered it in 1949. The dual to the Császár polyhedron, the Szilassi polyhedron, was discovered later, in 1977, by Lajos Szilassi; it has 14 vertices, 21 edges, and seven hexagonal faces, each sharing an edge with every other face. Like the Császár polyhedron, the Szilassi polyhedron has the topology of a torus.

There are other known polyhedra such as the Schönhardt polyhedron for which there are no interior diagonals (that is, all diagonals are outside the polyhedron) as well as non-manifold surfaces with no diagonals (Szabó 1984, 2009).

References[]

• Császár, A. (1949), "A polyhedron without diagonals" (PDF), Acta Sci. Math. Szeged, 13: 140–142, archived from the original (PDF) on 2017-09-18.
• Gardner, Martin (1988), Time Travel and Other Mathematical Bewilderments, W. H. Freeman and Company, pp. 139–152, Bibcode:1988ttom.book.....G, ISBN 0-7167-1924-X
• Gardner, Martin (1992), Fractal Music, Hypercards and More: Mathematical Recreations from Scientific American, W. H. Freeman and Company, pp. 118–120, ISBN 0-7167-2188-0
• Lutz, Frank H. (2001), "Császár's Torus", Electronic Geometry Models: 2001.02.069.
• Szabó, Sándor (1984), "Polyhedra without diagonals", Periodica Mathematica Hungarica, 15 (1): 41–49, doi:10.1007/BF02109370, S2CID 189834222.
• Szabó, Sándor (2009), "Polyhedra without diagonals II", Periodica Mathematica Hungarica, 58 (2): 181–187, doi:10.1007/s10998-009-10181-x, S2CID 45731540.
• Ziegler, Günter M. (2008), "Polyhedral Surfaces of High Genus", in Bobenko, A. I.; Schröder, P.; Sullivan, J. M.; Ziegler, G. M. (eds.), Discrete Differential Geometry, Oberwolfach Seminars, vol. 38, Springer-Verlag, pp. 191–213, arXiv:math.MG/0412093, doi:10.1007/978-3-7643-8621-4_10, ISBN 978-3-7643-8620-7, S2CID 15911143.

External links[]

• Weisstein, Eric W. "Csaszar Polyhedron". MathWorld.
• Császár’s polyhedron in virtual reality in NeoTrie VR.