Equalities that involve trigonometric functions
In mathematics , trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined. Geometrically, these are identities involving certain functions of one or more angles . They are distinct from triangle identities , which are identities potentially involving angles but also involving side lengths or other lengths of a triangle .
These identities are useful whenever expressions involving trigonometric functions need to be simplified. An important application is the integration of non-trigonometric functions: a common technique involves first using the substitution rule with a trigonometric function , and then simplifying the resulting integral with a trigonometric identity.
Notation [ ] Angles [ ]
Signs of trigonometric functions in each quadrant. The mnemonic "
All S cience
T eachers (are)
C razy" lists the basic functions ('
All' ,
s in,
t an,
c os) which are positive from quadrants I to IV.
[1] This is a variation on the mnemonic "
All Students Take Calculus ".
This article uses Greek letters such as alpha (
α
{\displaystyle \alpha }
beta (
β
{\displaystyle \beta }
gamma (
γ
{\displaystyle \gamma }
theta (
θ
{\displaystyle \theta }
angles . Several different units of angle measure are widely used, including degree , radian , and gradian (gons ):
1 complete rotation (turn )
=
360
∘
=
2
π
radians
=
400
gons.
{\displaystyle =360^{\circ }=2\pi {\text{ radians }}=400{\text{ gons. }}}
If not specifically annotated by (
∘
{\displaystyle {}^{\circ }}
g
{\displaystyle ^{\mathrm {g} }}
The following table shows for some common angles their conversions and the values of the basic trigonometric functions:
Conversions of common angles
Turn
Degree
Radian
Gradian
Sine
Cosine
Tangent
0
{\displaystyle 0}
0
∘
{\displaystyle 0^{\circ }}
0
{\displaystyle 0}
0
g
{\displaystyle 0^{\mathrm {g} }}
0
{\displaystyle 0}
1
{\displaystyle 1}
0
{\displaystyle 0}
1
12
{\displaystyle {\frac {1}{12}}}
30
∘
{\displaystyle 30^{\circ }}
π
6
{\displaystyle {\frac {\pi }{6}}}
33
1
3
g
{\displaystyle 33{\frac {1}{3}}^{\mathrm {g} }}
1
2
{\displaystyle {\frac {1}{2}}}
3
2
{\displaystyle {\frac {\sqrt {3}}{2}}}
3
3
{\displaystyle {\frac {\sqrt {3}}{3}}}
1
8
{\displaystyle {\frac {1}{8}}}
45
∘
{\displaystyle 45^{\circ }}
π
4
{\displaystyle {\frac {\pi }{4}}}
50
g
{\displaystyle 50^{\mathrm {g} }}
2
2
{\displaystyle {\frac {\sqrt {2}}{2}}}
2
2
{\displaystyle {\frac {\sqrt {2}}{2}}}
1
{\displaystyle 1}
1
6
{\displaystyle {\frac {1}{6}}}
60
∘
{\displaystyle 60^{\circ }}
π
3
{\displaystyle {\frac {\pi }{3}}}
66
2
3
g
{\displaystyle 66{\frac {2}{3}}^{\mathrm {g} }}
3
2
{\displaystyle {\frac {\sqrt {3}}{2}}}
1
2
{\displaystyle {\frac {1}{2}}}
3
{\displaystyle {\sqrt {3}}}
1
4
{\displaystyle {\frac {1}{4}}}
90
∘
{\displaystyle 90^{\circ }}
π
2
{\displaystyle {\frac {\pi }{2}}}
100
g
{\displaystyle 100^{\mathrm {g} }}
1
{\displaystyle 1}
0
{\displaystyle 0}
Undefined
1
3
{\displaystyle {\frac {1}{3}}}
120
∘
{\displaystyle 120^{\circ }}
2
π
3
{\displaystyle {\frac {2\pi }{3}}}
133
1
3
g
{\displaystyle 133{\frac {1}{3}}^{\mathrm {g} }}
3
2
{\displaystyle {\frac {\sqrt {3}}{2}}}
−
1
2
{\displaystyle -{\frac {1}{2}}}
−
3
{\displaystyle -{\sqrt {3}}}
3
8
{\displaystyle {\frac {3}{8}}}
135
∘
{\displaystyle 135^{\circ }}
3
π
4
{\displaystyle {\frac {3\pi }{4}}}
150
g
{\displaystyle 150^{\mathrm {g} }}
2
2
{\displaystyle {\frac {\sqrt {2}}{2}}}
−
2
2
{\displaystyle -{\frac {\sqrt {2}}{2}}}
−
1
{\displaystyle -1}
5
12
{\displaystyle {\frac {5}{12}}}
150
∘
{\displaystyle 150^{\circ }}
5
π
6
{\displaystyle {\frac {5\pi }{6}}}
166
2
3
g
{\displaystyle 166{\frac {2}{3}}^{\mathrm {g} }}
1
2
{\displaystyle {\frac {1}{2}}}
−
3
2
{\displaystyle -{\frac {\sqrt {3}}{2}}}
−
3
3
{\displaystyle -{\frac {\sqrt {3}}{3}}}
1
2
{\displaystyle {\frac {1}{2}}}
180
∘
{\displaystyle 180^{\circ }}
π
{\displaystyle \pi }
200
g
{\displaystyle 200^{\mathrm {g} }}
0
{\displaystyle 0}
−
1
{\displaystyle -1}
0
{\displaystyle 0}
7
12
{\displaystyle {\frac {7}{12}}}
210
∘
{\displaystyle 210^{\circ }}
7
π
6
{\displaystyle {\frac {7\pi }{6}}}
233
1
3
g
{\displaystyle 233{\frac {1}{3}}^{\mathrm {g} }}
−
1
2
{\displaystyle -{\frac {1}{2}}}
−
3
2
{\displaystyle -{\frac {\sqrt {3}}{2}}}
3
3
{\displaystyle {\frac {\sqrt {3}}{3}}}
5
8
{\displaystyle {\dfrac {5}{8}}}
225
∘
{\displaystyle 225^{\circ }}
5
π
4
{\displaystyle {\dfrac {5\pi }{4}}}
250
g
{\displaystyle 250^{\mathrm {g} }}
−
2
2
{\displaystyle -{\dfrac {\sqrt {2}}{2}}}
−
2
2
{\displaystyle -{\dfrac {\sqrt {2}}{2}}}
1
{\displaystyle 1}
2
3
{\displaystyle {\frac {2}{3}}}
240
∘
{\displaystyle 240^{\circ }}
4
π
3
{\displaystyle {\frac {4\pi }{3}}}
266
2
3
g
{\displaystyle 266{\frac {2}{3}}^{\mathrm {g} }}
−
3
2
{\displaystyle -{\frac {\sqrt {3}}{2}}}
−
1
2
{\displaystyle -{\frac {1}{2}}}
3
{\displaystyle {\sqrt {3}}}
3
4
{\displaystyle {\frac {3}{4}}}
270
∘
{\displaystyle 270^{\circ }}
3
π
2
{\displaystyle {\frac {3\pi }{2}}}
300
g
{\displaystyle 300^{\mathrm {g} }}
−
1
{\displaystyle -1}
0
{\displaystyle 0}
Undefined
5
6
{\displaystyle {\frac {5}{6}}}
300
∘
{\displaystyle 300^{\circ }}
5
π
3
{\displaystyle {\frac {5\pi }{3}}}
333
1
3
g
{\displaystyle 333{\frac {1}{3}}^{\mathrm {g} }}
−
3
2
{\displaystyle -{\frac {\sqrt {3}}{2}}}
1
2
{\displaystyle {\frac {1}{2}}}
−
3
{\displaystyle -{\sqrt {3}}}
7
8
{\displaystyle {\frac {7}{8}}}
315
∘
{\displaystyle 315^{\circ }}
7
π
4
{\displaystyle {\frac {7\pi }{4}}}
350
g
{\displaystyle 350^{\mathrm {g} }}
−
2
2
{\displaystyle -{\frac {\sqrt {2}}{2}}}
2
2
{\displaystyle {\frac {\sqrt {2}}{2}}}
−
1
{\displaystyle -1}
11
12
{\displaystyle {\frac {11}{12}}}
330
∘
{\displaystyle 330^{\circ }}
11
π
6
{\displaystyle {\frac {11\pi }{6}}}
366
2
3
g
{\displaystyle 366{\frac {2}{3}}^{\mathrm {g} }}
−
1
2
{\displaystyle -{\frac {1}{2}}}
3
2
{\displaystyle {\frac {\sqrt {3}}{2}}}
−
3
3
{\displaystyle -{\frac {\sqrt {3}}{3}}}
1
{\displaystyle 1}
360
∘
{\displaystyle 360^{\circ }}
2
π
{\displaystyle 2\pi }
400
g
{\displaystyle 400^{\mathrm {g} }}
0
{\displaystyle 0}
1
{\displaystyle 1}
0
{\displaystyle 0}
Results for other angles can be found at Trigonometric constants expressed in real radicals . Per Niven's theorem ,
(
0
,
30
,
90
,
150
,
180
,
210
,
270
,
330
,
360
)
{\displaystyle (0,\;30,\;90,\;150,\;180,\;210,\;270,\;330,\;360)}
[2] [3] [4]
π
{\displaystyle \pi }
0
,
π
/
6
,
π
/
2
,
5
π
/
6
,
π
,
7
π
/
6
,
3
π
/
2
,
11
π
/
6
(
,
2
π
)
.
{\displaystyle 0,\pi /6,\pi /2,5\pi /6,\pi ,7\pi /6,3\pi /2,11\pi /6(,2\pi ).}
Trigonometric functions [ ]
Plot of the six trigonometric functions, the unit circle, and a line for the angle
θ
=
0.7
{\displaystyle \theta =0.7}
radians. The points labelled
1 ,
Sec(θ) ,
Csc(θ) represent the length of the line segment from the origin to that point.
Sin(θ) ,
Tan(θ) , and
1 are the heights to the line starting from the
x
{\displaystyle x}
-axis, while
Cos(θ) ,
1 , and
Cot(θ) are lengths along the
x
{\displaystyle x}
-axis starting from the origin.
The functions sine , cosine and tangent of an angle are sometimes referred to as the primary or basic trigonometric functions. Their usual abbreviations are
sin
(
θ
)
,
cos
(
θ
)
,
{\displaystyle \sin(\theta ),\cos(\theta ),}
tan
(
θ
)
,
{\displaystyle \tan(\theta ),}
θ
{\displaystyle \theta }
sin
θ
{\displaystyle \sin \theta }
cos
θ
,
{\displaystyle \cos \theta ,}
The sine of an angle is defined, in the context of a right triangle , as the ratio of the length of the side that is opposite to the angle divided by the length of the longest side of the triangle (the hypotenuse ).
sin
θ
=
opposite
hypotenuse
.
{\displaystyle \sin \theta ={\frac {\text{opposite}}{\text{hypotenuse}}}.}
The cosine of an angle in this context is the ratio of the length of the side that is adjacent to the angle divided by the length of the hypotenuse.
cos
θ
=
adjacent
hypotenuse
.
{\displaystyle \cos \theta ={\frac {\text{adjacent}}{\text{hypotenuse}}}.}
The tangent of an angle in this context is the ratio of the length of the side that is opposite to the angle divided by the length of the side that is adjacent to the angle. This is the same as the ratio of the sine to the cosine of this angle, as can be seen by substituting the definitions of
sin
{\displaystyle \sin }
cos
{\displaystyle \cos }
tan
θ
=
sin
θ
cos
θ
=
opposite
adjacent
.
{\displaystyle \tan \theta ={\frac {\sin \theta }{\cos \theta }}={\frac {\text{opposite}}{\text{adjacent}}}.}
The remaining trigonometric functions secant (
sec
{\displaystyle \sec }
csc
{\displaystyle \csc }
cot
{\displaystyle \cot }
reciprocal functions of cosine, sine, and tangent, respectively. Rarely, these are called the secondary trigonometric functions:
sec
θ
=
1
cos
θ
,
csc
θ
=
1
sin
θ
,
cot
θ
=
1
tan
θ
=
cos
θ
sin
θ
.
{\displaystyle \sec \theta ={\frac {1}{\cos \theta }},\quad \csc \theta ={\frac {1}{\sin \theta }},\quad \cot \theta ={\frac {1}{\tan \theta }}={\frac {\cos \theta }{\sin \theta }}.}
These definitions are sometimes referred to as ratio identities .
Other functions [ ]
sgn
x
{\displaystyle \operatorname {sgn} x}
sign function , which is defined as:
sgn
(
x
)
=
{
−
1
if
x
<
0
,
0
if
x
=
0
,
1
if
x
>
0.
{\displaystyle \operatorname {sgn}(x)={\begin{cases}-1&{\text{if }}x<0,\\0&{\text{if }}x=0,\\1&{\text{if }}x>0.\end{cases}}}
Inverse functions [ ] The inverse trigonometric functions are partial inverse functions for the trigonometric functions. For example, the inverse function for the sine, known as the inverse sine (
sin
−
1
{\displaystyle \sin ^{-1}}
arcsine (arcsin or asin ), satisfies
sin
(
arcsin
x
)
=
x
for
|
x
|
≤
1
{\displaystyle \sin(\arcsin x)=x\quad {\text{for}}\quad |x|\leq 1}
and
arcsin
(
sin
x
)
=
x
for
|
x
|
≤
π
2
.
{\displaystyle \arcsin(\sin x)=x\quad {\text{for}}\quad |x|\leq {\frac {\pi }{2}}.}
This article will denote the inverse of a trigonometric function by prefixing its name with "
arc
{\displaystyle \operatorname {arc} }
The table below displays names and domains of the inverse trigonometric functions along with the range of their usual principal values in radians .
Original
Abbreviation
Domain
Image /range
Inverse
Domain of
Range of usualprincipal values of inverse
sine
sin
{\displaystyle \sin }
:
{\displaystyle :}
R
{\displaystyle \mathbb {R} }
→
{\displaystyle \to }
[
−
1
,
1
]
{\displaystyle [-1,1]}
arcsin
{\displaystyle \arcsin }
:
{\displaystyle :}
[
−
1
,
1
]
{\displaystyle [-1,1]}
→
{\displaystyle \to }
[
−
π
2
,
π
2
]
{\displaystyle \left[-{\frac {\pi }{2}},{\frac {\pi }{2}}\right]}
cosine
cos
{\displaystyle \cos }
:
{\displaystyle :}
R
{\displaystyle \mathbb {R} }
→
{\displaystyle \to }
[
−
1
,
1
]
{\displaystyle [-1,1]}
arccos
{\displaystyle \arccos }
:
{\displaystyle :}
[
−
1
,
1
]
{\displaystyle [-1,1]}
→
{\displaystyle \to }
[
0
,
π
]
{\displaystyle [0,\pi ]}
tangent
tan
{\displaystyle \tan }
:
{\displaystyle :}
π
Z
+
(
−
π
2
,
π
2
)
{\displaystyle \pi \mathbb {Z} +\left(-{\frac {\pi }{2}},{\frac {\pi }{2}}\right)}
→
{\displaystyle \to }
R
{\displaystyle \mathbb {R} }
arctan
{\displaystyle \arctan }
:
{\displaystyle :}
R
{\displaystyle \mathbb {R} }
→
{\displaystyle \to }
(
−
π
2
,
π
2
)
{\displaystyle \left(-{\frac {\pi }{2}},{\frac {\pi }{2}}\right)}
cotangent
cot
{\displaystyle \cot }
:
{\displaystyle :}
π
Z
+
(
0
,
π
)
{\displaystyle \pi \mathbb {Z} +(0,\pi )}
→
{\displaystyle \to }
R
{\displaystyle \mathbb {R} }
arccot
{\displaystyle \operatorname {arccot} }
:
{\displaystyle :}
R
{\displaystyle \mathbb {R} }
→
{\displaystyle \to }
(
0
,
π
)
{\displaystyle (0,\pi )}
secant
sec
{\displaystyle \sec }
:
{\displaystyle :}
π
Z
+
(
−
π
2
,
π
2
)
{\displaystyle \pi \mathbb {Z} +\left(-{\frac {\pi }{2}},{\frac {\pi }{2}}\right)}
→
{\displaystyle \to }
(
−
∞
,
−
1
]
∪
[
1
,
∞
)
{\displaystyle (-\infty ,-1]\cup [1,\infty )}
arcsec
{\displaystyle \operatorname {arcsec} }
:
{\displaystyle :}
(
−
∞
,
−
1
]
∪
[
1
,
∞
)
{\displaystyle (-\infty ,-1]\cup [1,\infty )}
→
{\displaystyle \to }
[
0
,
π
2
)
∪
(
π
2
,
π
]
{\displaystyle \left[0,{\frac {\pi }{2}}\right)\cup \left({\frac {\pi }{2}},\pi \right]}
cosecant
csc
{\displaystyle \csc }
:
{\displaystyle :}
π
Z
+
(
0
,
π
)
{\displaystyle \pi \mathbb {Z} +(0,\pi )}
→
{\displaystyle \to }
(
−
∞
,
−
1
]
∪
[
1
,
∞
)
{\displaystyle (-\infty ,-1]\cup [1,\infty )}
arccsc
{\displaystyle \operatorname {arccsc} }
:
{\displaystyle :}
(
−
∞
,
−
1
]
∪
[
1
,
∞
)
{\displaystyle (-\infty ,-1]\cup [1,\infty )}
→
{\displaystyle \to }
[
−
π
2
,
0
)
∪
(
0
,
π
2
]
{\displaystyle \left[-{\frac {\pi }{2}},0\right)\cup \left(0,{\frac {\pi }{2}}\right]}
The symbol
R
=
(
−
∞
,
∞
)
{\displaystyle \mathbb {R} =(-\infty ,\infty )}
real numbers and
Z
=
{
…
,
−
2
,
−
1
,
0
,
1
,
2
,
…
}
{\displaystyle \mathbb {Z} =\{\ldots ,\,-2,\,-1,\,0,\,1,\,2,\,\ldots \}}
integers .
The set of all integer multiples of
π
{\displaystyle \pi }
π
Z
:=
{
π
n
:
n
∈
Z
}
=
{
…
,
−
2
π
,
−
π
,
0
,
π
,
2
π
,
3
π
,
4
π
,
…
}
=
{
θ
∈
R
:
sin
θ
=
0
}
.
{\displaystyle \pi \mathbb {Z} ~:=~\{\pi n\;:\;n\in \mathbb {Z} \}~=~\{\ldots ,\,-2\pi ,\,-\pi ,\,0,\,\pi ,\,2\pi ,\,3\pi ,\,4\pi ,\,\ldots \}~=~\{\theta \in \mathbb {R} \;:\;\sin \theta =0\}.}
The
Minkowski sum notation
π
Z
+
(
0
,
π
)
{\displaystyle \pi \mathbb {Z} +(0,\pi )}
means
π
Z
+
(
0
,
π
)
=
⋯
∪
(
−
2
π
,
−
π
)
∪
(
−
π
,
0
)
∪
(
0
,
π
)
∪
(
π
,
2
π
)
∪
(
2
π
,
3
π
)
∪
(
3
π
,
4
π
)
∪
⋯
=
R
∖
π
Z
{\displaystyle {\begin{alignedat}{4}\pi \mathbb {Z} +(0,\pi )&~=~\cdots \cup (-2\pi ,-\pi )\cup (-\pi ,0)\cup (0,\pi )\cup (\pi ,2\pi )\cup (2\pi ,3\pi )\cup (3\pi ,4\pi )\cup \cdots \\&~=~\mathbb {R} \setminus \pi \mathbb {Z} \\\end{alignedat}}}
where
∖
{\displaystyle \,\setminus \,}
denotes
set subtraction . In other words, the domain of
cot
{\displaystyle \,\cot \,}
and
csc
{\displaystyle \,\csc \,}
is the set
R
∖
π
Z
{\displaystyle \mathbb {R} \setminus \pi \mathbb {Z} }
of all real numbers that are
not of the form
π
n
{\displaystyle \pi n}
for some integer
n
.
{\displaystyle n.}
Similarly, the domain of
tan
{\displaystyle \,\tan \,}
sec
{\displaystyle \,\sec \,}
π
Z
+
(
−
π
2
,
π
2
)
=
⋯
∪
(
−
3
π
2
,
−
π
2
)
∪
(
−
π
2
,
π
2
)
∪
(
π
2
,
3
π
2
)
∪
(
3
π
2
,
5
π
2
)
∪
(
5
π
2
,
7
π
2
)
∪
⋯
=
R
∖
(
π
2
+
π
Z
)
{\displaystyle {\begin{alignedat}{4}\pi \mathbb {Z} +\left(-{\frac {\pi }{2}},{\frac {\pi }{2}}\right)&~=~\cdots \cup \left(-{\frac {3\pi }{2}},-{\frac {\pi }{2}}\right)\cup \left(-{\frac {\pi }{2}},{\frac {\pi }{2}}\right)\cup \left({\frac {\pi }{2}},{\frac {3\pi }{2}}\right)\cup \left({\frac {3\pi }{2}},{\frac {5\pi }{2}}\right)\cup \left({\frac {5\pi }{2}},{\frac {7\pi }{2}}\right)\cup \cdots \\&~=~\mathbb {R} \setminus \left({\frac {\pi }{2}}+\pi \mathbb {Z} \right)\\\end{alignedat}}}
where
R
∖
(
π
2
+
π
Z
)
{\displaystyle \mathbb {R} \setminus \left({\frac {\pi }{2}}+\pi \mathbb {Z} \right)}
is the set of all real numbers that do
not belong to the set
π
2
+
π
Z
:=
{
π
2
+
π
n
:
n
∈
Z
}
=
{
…
,
−
3
π
2
,
−
π
2
,
π
2
,
3
π
2
,
5
π
2
,
7
π
2
,
…
}
=
{
θ
∈
R
:
cos
θ
=
0
}
;
{\displaystyle {\frac {\pi }{2}}+\pi \mathbb {Z} ~:=~\left\{{\frac {\pi }{2}}+\pi n:n\in \mathbb {Z} \right\}=\left\{\ldots ,\,-{\frac {3\pi }{2}},\,-{\frac {\pi }{2}},\,{\frac {\pi }{2}},\,{\frac {3\pi }{2}},\,{\frac {5\pi }{2}},\,{\frac {7\pi }{2}},\,\ldots \right\}~=~\{\theta \in \mathbb {R} \;:\;\cos \theta =0\};}
said differently, the domain of
tan
{\displaystyle \,\tan \,}
and
sec
{\displaystyle \,\sec \,}
is the set of all real numbers that are
not of the form
π
2
+
π
n
{\displaystyle {\frac {\pi }{2}}+\pi n}
for some integer
n
.
{\displaystyle n.}
These inverse trigonometric functions are related to one another by the formulas:
π
2
=
arcsin
(
x
)
+
arccos
(
x
)
=
arctan
(
r
)
+
arccot
(
r
)
=
arcsec
(
s
)
+
arccsc
(
s
)
π
=
arccos
(
x
)
+
arccos
(
−
x
)
=
arccot
(
r
)
+
arccot
(
−
r
)
=
arcsec
(
s
)
+
arcsec
(
−
s
)
0
=
arcsin
(
x
)
+
arcsin
(
−
x
)
=
arctan
(
r
)
+
arctan
(
−
r
)
=
arccsc
(
s
)
+
arccsc
(
−
s
)
{\displaystyle {\begin{alignedat}{9}{\frac {\pi }{2}}~&=~\arcsin(x)&&+\arccos(x)~&&=~\arctan(r)&&+\operatorname {arccot}(r)~&&=~\operatorname {arcsec}(s)&&+\operatorname {arccsc}(s)\\[0.4ex]\pi ~&=~\arccos(x)&&+\arccos(-x)~&&=~\operatorname {arccot}(r)&&+\operatorname {arccot}(-r)~&&=~\operatorname {arcsec}(s)&&+\operatorname {arcsec}(-s)\\[0.4ex]0~&=~\arcsin(x)&&+\arcsin(-x)~&&=~\arctan(r)&&+\arctan(-r)~&&=~\operatorname {arccsc}(s)&&+\operatorname {arccsc}(-s)\\[1.0ex]\end{alignedat}}}
which hold whenever they are well-defined (that is, whenever
x
,
r
,
s
,
−
x
,
−
r
,
and
−
s
{\displaystyle x,r,s,-x,-r,{\text{ and }}-s}
are in the domains of the relevant functions).
Solutions to elementary trigonometric equations [ ] The following table shows how inverse trigonometric functions may be used to solve equalities involving the six standard trigonometric functions.
It is assumed that the given values
θ
,
r
,
s
,
x
,
{\displaystyle \theta ,r,s,x,}
y
{\displaystyle y}
well-defined .
In the table below, "for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
integer
k
.
{\displaystyle k.}
Equation
if and only if Solution
where...
sin
θ
=
y
{\displaystyle \sin \theta =y}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
(
−
1
)
k
{\displaystyle (-1)^{k}}
arcsin
(
y
)
{\displaystyle \arcsin(y)}
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
csc
θ
=
r
{\displaystyle \csc \theta =r}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
(
−
1
)
k
{\displaystyle (-1)^{k}}
arccsc
(
r
)
{\displaystyle \operatorname {arccsc}(r)}
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
cos
θ
=
x
{\displaystyle \cos \theta =x}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
±
{\displaystyle \pm \,}
arccos
(
x
)
{\displaystyle \arccos(x)}
+
{\displaystyle +}
2
{\displaystyle 2}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
sec
θ
=
r
{\displaystyle \sec \theta =r}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
±
{\displaystyle \pm \,}
arcsec
(
r
)
{\displaystyle \operatorname {arcsec}(r)}
+
{\displaystyle +}
2
{\displaystyle 2}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
tan
θ
=
s
{\displaystyle \tan \theta =s}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
arctan
(
s
)
{\displaystyle \arctan(s)}
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
cot
θ
=
r
{\displaystyle \cot \theta =r}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =\,}
arccot
(
r
)
{\displaystyle \operatorname {arccot}(r)}
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
For example, if
cos
θ
=
−
1
{\displaystyle \cos \theta =-1}
θ
=
π
+
2
π
k
=
−
π
+
2
π
(
1
+
k
)
{\displaystyle \theta =\pi +2\pi k=-\pi +2\pi (1+k)}
k
∈
Z
.
{\displaystyle k\in \mathbb {Z} .}
sin
θ
=
±
1
{\displaystyle \sin \theta =\pm 1}
θ
=
π
2
+
π
k
=
−
π
2
+
π
(
k
+
1
)
{\displaystyle \theta ={\frac {\pi }{2}}+\pi k=-{\frac {\pi }{2}}+\pi (k+1)}
k
∈
Z
,
{\displaystyle k\in \mathbb {Z} ,}
k
{\displaystyle k}
sin
θ
=
1
{\displaystyle \sin \theta =1}
sin
θ
=
−
1.
{\displaystyle \sin \theta =-1.}
sec
θ
=
−
1
{\displaystyle \sec \theta =-1}
csc
θ
=
±
1
{\displaystyle \csc \theta =\pm 1}
cos
θ
=
−
1
{\displaystyle \cos \theta =-1}
sin
θ
=
±
1
,
{\displaystyle \sin \theta =\pm 1,}
except for those just solved (i.e. except for
sin
{\displaystyle \sin }
csc
θ
=
±
1
{\displaystyle \csc \theta =\pm 1}
cos
{\displaystyle \cos }
sec
θ
=
−
1
{\displaystyle \sec \theta =-1}
r
,
s
,
x
,
{\displaystyle r,s,x,}
y
,
{\displaystyle y,}
k
{\displaystyle k}
θ
.
{\displaystyle \theta .}
The table below shows how two angles
θ
{\displaystyle \theta }
φ
{\displaystyle \varphi }
Equation
if and only if Solution
where...
Also a solution to
sin
θ
{\displaystyle \sin \theta }
=
{\displaystyle =}
sin
φ
{\displaystyle \sin \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
(
−
1
)
k
{\displaystyle (-1)^{k}}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
csc
θ
=
csc
φ
{\displaystyle \csc \theta =\csc \varphi }
cos
θ
{\displaystyle \cos \theta }
=
{\displaystyle =}
cos
φ
{\displaystyle \cos \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
±
{\displaystyle \pm \,}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
2
{\displaystyle 2}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
sec
θ
=
sec
φ
{\displaystyle \sec \theta =\sec \varphi }
tan
θ
{\displaystyle \tan \theta }
=
{\displaystyle =}
tan
φ
{\displaystyle \tan \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
cot
θ
=
cot
φ
{\displaystyle \cot \theta =\cot \varphi }
−
sin
θ
{\displaystyle -\sin \theta }
=
{\displaystyle =}
sin
φ
{\displaystyle \sin \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
(
−
1
)
k
+
1
{\displaystyle (-1)^{k+1}}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
−
csc
θ
=
csc
φ
{\displaystyle -\csc \theta =\csc \varphi }
−
cos
θ
{\displaystyle -\cos \theta }
=
{\displaystyle =}
cos
φ
{\displaystyle \cos \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
±
{\displaystyle \pm \,}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
2
{\displaystyle 2}
π
k
{\displaystyle \pi k}
+
π
{\displaystyle +\,\;\pi }
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
−
sec
θ
=
sec
φ
{\displaystyle -\sec \theta =\sec \varphi }
−
tan
θ
{\displaystyle -\tan \theta }
=
{\displaystyle =}
tan
φ
{\displaystyle \tan \varphi }
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
−
{\displaystyle -}
φ
{\displaystyle \varphi }
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
−
cot
θ
=
cot
φ
{\displaystyle -\cot \theta =\cot \varphi }
|
sin
θ
|
{\displaystyle |\sin \theta |}
=
{\displaystyle =}
|
sin
φ
|
{\displaystyle |\sin \varphi |}
⟺
{\displaystyle \iff }
θ
=
{\displaystyle \theta =}
±
{\displaystyle \pm }
φ
{\displaystyle \varphi }
+
{\displaystyle +}
π
k
{\displaystyle \pi k}
for some
k
∈
Z
{\displaystyle k\in \mathbb {Z} }
|
tan
θ
|
=
|
tan
φ
|
{\displaystyle |\tan \theta |=|\tan \varphi |}
⇕
|
csc
θ
|
=
|
csc
φ
|
{\displaystyle |\csc \theta |=|\csc \varphi |}
|
cos
θ
|
{\displaystyle |\cos \theta |}
=
{\displaystyle =}
|
cos
φ
|
{\displaystyle |\cos \varphi |}
|
sec
θ
|
=
|
sec
φ
|
{\displaystyle |\sec \theta |=|\sec \varphi |}
|
cot
θ
|
=
|
cot
φ
|
{\displaystyle |\cot \theta |=|\cot \varphi |}
Pythagorean identities [ ] In trigonometry, the basic relationship between the sine and the cosine is given by the Pythagorean identity:
sin
2
θ
+
cos
2
θ
=
1
,
{\displaystyle \sin ^{2}\theta +\cos ^{2}\theta =1,}
where
sin
2
θ
{\displaystyle \sin ^{2}\theta }
means
(
sin
θ
)
2
{\displaystyle (\sin \theta )^{2}}
and
cos
2
θ
{\displaystyle \cos ^{2}\theta }
means
(
cos
θ
)
2
.
{\displaystyle (\cos \theta )^{2}.}
This can be viewed as a version of the Pythagorean theorem , and follows from the equation
x
2
+
y
2
=
1
{\displaystyle x^{2}+y^{2}=1}
unit circle . This equation can be solved for either the sine or the cosine:
sin
θ
=
±
1
−
cos
2
θ
,
cos
θ
=
±
1
−
sin
2
θ
.
{\displaystyle {\begin{aligned}\sin \theta &=\pm {\sqrt {1-\cos ^{2}\theta }},\\\cos \theta &=\pm {\sqrt {1-\sin ^{2}\theta }}.\end{aligned}}}
where the sign depends on the quadrant of
θ
.
{\displaystyle \theta .}
Dividing this identity by either
sin
2
θ
{\displaystyle \sin ^{2}\theta }
cos
2
θ
{\displaystyle \cos ^{2}\theta }
1
+
cot
2
θ
=
csc
2
θ
and
tan
2
θ
+
1
=
sec
2
θ
.
{\displaystyle 1+\cot ^{2}\theta =\csc ^{2}\theta \quad {\text{and}}\quad \tan ^{2}\theta +1=\sec ^{2}\theta .}
Using these identities together with the ratio identities, it is possible to express any trigonometric function in terms of any other (up to a plus or minus sign):
Each trigonometric function in terms of each of the other five.[5]
in terms of
sin
θ
{\displaystyle \sin \theta }
cos
θ
{\displaystyle \cos \theta }
tan
θ
{\displaystyle \tan \theta }
csc
θ
{\displaystyle \csc \theta }
sec
θ
{\displaystyle \sec \theta }
cot
θ
{\displaystyle \cot \theta }
sin
θ
=
{\displaystyle \sin \theta =}
sin
θ
{\displaystyle \sin \theta }
±
1
−
cos
2
θ
{\displaystyle \pm {\sqrt {1-\cos ^{2}\theta }}}
±
tan
θ
1
+
tan
2
θ
{\displaystyle \pm {\frac {\tan \theta }{\sqrt {1+\tan ^{2}\theta }}}}
1
csc
θ
{\displaystyle {\frac {1}{\csc \theta }}}
±
sec
2
θ
−
1
sec
θ
{\displaystyle \pm {\frac {\sqrt {\sec ^{2}\theta -1}}{\sec \theta }}}
±
1
1
+
cot
2
θ
{\displaystyle \pm {\frac {1}{\sqrt {1+\cot ^{2}\theta }}}}
cos
θ
=
{\displaystyle \cos \theta =}
±
1
−
sin
2
θ
{\displaystyle \pm {\sqrt {1-\sin ^{2}\theta }}}
cos
θ
{\displaystyle \cos \theta }
±
1
1
+
tan
2
θ
{\displaystyle \pm {\frac {1}{\sqrt {1+\tan ^{2}\theta }}}}
±
csc
2
θ
−
1
csc
θ
{\displaystyle \pm {\frac {\sqrt {\csc ^{2}\theta -1}}{\csc \theta }}}
1
sec
θ
{\displaystyle {\frac {1}{\sec \theta }}}
±
cot
θ
1
+
cot
2
θ
{\displaystyle \pm {\frac {\cot \theta }{\sqrt {1+\cot ^{2}\theta }}}}
tan
θ
=
{\displaystyle \tan \theta =}
±
sin
θ
1
−
sin
2
θ
{\displaystyle \pm {\frac {\sin \theta }{\sqrt {1-\sin ^{2}\theta }}}}
±
1
−
cos
2
θ
cos
θ
{\displaystyle \pm {\frac {\sqrt {1-\cos ^{2}\theta }}{\cos \theta }}}
tan
θ
{\displaystyle \tan \theta }
±
1
csc
2
θ
−
1
{\displaystyle \pm {\frac {1}{\sqrt {\csc ^{2}\theta -1}}}}
±
sec
2
θ
−
1
{\displaystyle \pm {\sqrt {\sec ^{2}\theta -1}}}
1
cot
θ
{\displaystyle {\frac {1}{\cot \theta }}}
csc
θ
=
{\displaystyle \csc \theta =}
1
sin
θ
{\displaystyle {\frac {1}{\sin \theta }}}
±
1
1
−
cos
2
θ
{\displaystyle \pm {\frac {1}{\sqrt {1-\cos ^{2}\theta }}}}
±
1
+
tan
2
θ
tan
θ
{\displaystyle \pm {\frac {\sqrt {1+\tan ^{2}\theta }}{\tan \theta }}}
csc
θ
{\displaystyle \csc \theta }
±
sec
θ
sec
2
θ
−
1
{\displaystyle \pm {\frac {\sec \theta }{\sqrt {\sec ^{2}\theta -1}}}}
±
1
+
cot
2
θ
{\displaystyle \pm {\sqrt {1+\cot ^{2}\theta }}}
sec
θ
=
{\displaystyle \sec \theta =}
±
1
1
−
sin
2
θ
{\displaystyle \pm {\frac {1}{\sqrt {1-\sin ^{2}\theta }}}}
1
cos
θ
{\displaystyle {\frac {1}{\cos \theta }}}
±
1
+
tan
2
θ
{\displaystyle \pm {\sqrt {1+\tan ^{2}\theta }}}
±
csc
θ
csc
2
θ
−
1
{\displaystyle \pm {\frac {\csc \theta }{\sqrt {\csc ^{2}\theta -1}}}}
sec
θ
{\displaystyle \sec \theta }
±
1
+
cot
2
θ
cot
θ
{\displaystyle \pm {\frac {\sqrt {1+\cot ^{2}\theta }}{\cot \theta }}}
cot
θ
=
{\displaystyle \cot \theta =}
±
1
−
sin
2
θ
sin
θ
{\displaystyle \pm {\frac {\sqrt {1-\sin ^{2}\theta }}{\sin \theta }}}
±
cos
θ
1
−
cos
2
θ
{\displaystyle \pm {\frac {\cos \theta }{\sqrt {1-\cos ^{2}\theta }}}}
1
tan
θ
{\displaystyle {\frac {1}{\tan \theta }}}
±
csc
2
θ
−
1
{\displaystyle \pm {\sqrt {\csc ^{2}\theta -1}}}
±
1
sec
2
θ
−
1
{\displaystyle \pm {\frac {1}{\sqrt {\sec ^{2}\theta -1}}}}
cot
θ
{\displaystyle \cot \theta }
Historical shorthands [ ]
All the trigonometric functions of an angle
θ
{\displaystyle \theta }
can be constructed geometrically in terms of a unit circle centered at
O . Many of these terms are no longer in common use; however, this diagram is not exhaustive.
[clarification needed The versine , coversine , haversine , and exsecant were used in navigation. For example, the haversine formula was used to calculate the distance between two points on a sphere. They are rarely used today.
Name
Abbreviation
Value[6] [7]
(right) complementary angle, co-angle
co
θ
{\displaystyle \operatorname {co} \theta }
π
2
−
θ
{\displaystyle {\pi \over 2}-\theta }
versed sine, versine
versin
θ
{\displaystyle \operatorname {versin} \theta }
vers
θ
{\displaystyle \operatorname {vers} \theta }
ver
θ
{\displaystyle \operatorname {ver} \theta }
1
−
cos
θ
{\displaystyle 1-\cos \theta }
versed cosine, vercosine
vercosin
θ
{\displaystyle \operatorname {vercosin} \theta }
vercos
θ
{\displaystyle \operatorname {vercos} \theta }
vcs
θ
{\displaystyle \operatorname {vcs} \theta }
1
+
cos
θ
{\displaystyle 1+\cos \theta }
coversed sine, coversine
coversin
θ
{\displaystyle \operatorname {coversin} \theta }
covers
θ
{\displaystyle \operatorname {covers} \theta }
cvs
θ
{\displaystyle \operatorname {cvs} \theta }
1
−
sin
θ
{\displaystyle 1-\sin \theta }
coversed cosine, covercosine
covercosin
θ
{\displaystyle \operatorname {covercosin} \theta }
covercos
θ
{\displaystyle \operatorname {covercos} \theta }
cvc
θ
{\displaystyle \operatorname {cvc} \theta }
1
+
sin
θ
{\displaystyle 1+\sin \theta }
half versed sine, haversine
haversin
θ
{\displaystyle \operatorname {haversin} \theta }
hav
θ
{\displaystyle \operatorname {hav} \theta }
sem
θ
{\displaystyle \operatorname {sem} \theta }
1
−
cos
θ
2
{\displaystyle {\frac {1-\cos \theta }{2}}}
half versed cosine, havercosine
havercosin
θ
{\displaystyle \operatorname {havercosin} \theta }
havercos
θ
{\displaystyle \operatorname {havercos} \theta }
hvc
θ
{\displaystyle \operatorname {hvc} \theta }
1
+
cos
θ
2
{\displaystyle {\frac {1+\cos \theta }{2}}}
half coversed sine, hacoversine
hacoversin
θ
{\displaystyle \operatorname {hacoversin} \theta }
hacovers
θ
{\displaystyle \operatorname {hacovers} \theta }
hcv
θ
{\displaystyle \operatorname {hcv} \theta }
1
−
sin
θ
2
{\displaystyle {\frac {1-\sin \theta }{2}}}
half coversed cosine, hacovercosine
hacovercosin
θ
{\displaystyle \operatorname {hacovercosin} \theta }
hacovercos
θ
{\displaystyle \operatorname {hacovercos} \theta }
hcc
θ
{\displaystyle \operatorname {hcc} \theta }
1
+
sin
θ
2
{\displaystyle {\frac {1+\sin \theta }{2}}}
exterior secant, exsecant
exsec
θ
{\displaystyle \operatorname {exsec} \theta }
exs
θ
{\displaystyle \operatorname {exs} \theta }
sec
θ
−
1
{\displaystyle \sec \theta -1}
exterior cosecant, excosecant
excosec
θ
{\displaystyle \operatorname {excosec} \theta }
excsc
θ
{\displaystyle \operatorname {excsc} \theta }
exc
θ
{\displaystyle \operatorname {exc} \theta }
csc
θ
−
1
{\displaystyle \csc \theta -1}
chord
crd
θ
{\displaystyle \operatorname {crd} \theta }
2
sin
θ
2
{\displaystyle 2\sin {\frac {\theta }{2}}}
Reflections, shifts, and periodicity [ ] By examining the unit circle, one can establish the following properties of the trigonometric functions.
Reflections [ ]
Transformation of coordinates (
a ,
b ) when shifting the reflection angle
α
{\displaystyle \alpha }
in increments of
π
4
{\displaystyle {\frac {\pi }{4}}}
.
When the direction of a Euclidean vector is represented by an angle
θ
,
{\displaystyle \theta ,}
x
{\displaystyle x}
x
{\displaystyle x}
θ
{\displaystyle \theta }
α
,
{\displaystyle \alpha ,}
θ
′
{\displaystyle \theta ^{\prime }}
θ
′
=
2
α
−
θ
.
{\displaystyle \theta ^{\prime }=2\alpha -\theta .}
The values of the trigonometric functions of these angles
θ
,
θ
′
{\displaystyle \theta ,\;\theta ^{\prime }}
α
{\displaystyle \alpha }
reduction formulae .[8]
θ
{\displaystyle \theta }
α
=
0
{\displaystyle \alpha =0}
[9] odd/even identities
θ
{\displaystyle \theta }
α
=
π
4
{\displaystyle \alpha ={\frac {\pi }{4}}}
θ
{\displaystyle \theta }
α
=
π
2
{\displaystyle \alpha ={\frac {\pi }{2}}}
θ
{\displaystyle \theta }
α
=
3
π
4
{\displaystyle \alpha ={\frac {3\pi }{4}}}
θ
{\displaystyle \theta }
α
=
π
{\displaystyle \alpha =\pi }
compare to
α
=
0
{\displaystyle \alpha =0}
sin
(
−
θ
)
=
−
sin
θ
{\displaystyle \sin(-\theta )=-\sin \theta }
sin
(
π
2
−
θ
)
=
cos
θ
{\displaystyle \sin \left({\tfrac {\pi }{2}}-\theta \right)=\cos \theta }
sin
(
π
−
θ
)
=
+
sin
θ
{\displaystyle \sin(\pi -\theta )=+\sin \theta }
sin
(
3
π
2
−
θ
)
=
−
cos
θ
{\displaystyle \sin \left({\tfrac {3\pi }{2}}-\theta \right)=-\cos \theta }
sin
(
2
π
−
θ
)
=
−
sin
(
θ
)
=
sin
(
−
θ
)
{\displaystyle \sin(2\pi -\theta )=-\sin(\theta )=\sin(-\theta )}
cos
(
−
θ
)
=
+
cos
θ
{\displaystyle \cos(-\theta )=+\cos \theta }
cos
(
π
2
−
θ
)
=
sin
θ
{\displaystyle \cos \left({\tfrac {\pi }{2}}-\theta \right)=\sin \theta }
cos
(
π
−
θ
)
=
−
cos
θ
{\displaystyle \cos(\pi -\theta )=-\cos \theta }
cos
(
3
π
2
−
θ
)
=
−
sin
θ
{\displaystyle \cos \left({\tfrac {3\pi }{2}}-\theta \right)=-\sin \theta }
cos
(
2
π
−
θ
)
=
+
cos
(
θ
)
=
cos
(
−
θ
)
{\displaystyle \cos(2\pi -\theta )=+\cos(\theta )=\cos(-\theta )}
tan
(
−
θ
)
=
−
tan
θ
{\displaystyle \tan(-\theta )=-\tan \theta }
tan
(
π
2
−
θ
)
=
cot
θ
{\displaystyle \tan \left({\tfrac {\pi }{2}}-\theta \right)=\cot \theta }
tan
(
π
−
θ
)
=
−
tan
θ
{\displaystyle \tan(\pi -\theta )=-\tan \theta }
tan
(
3
π
2
−
θ
)
=
+
cot
θ
{\displaystyle \tan \left({\tfrac {3\pi }{2}}-\theta \right)=+\cot \theta }
tan
(
2
π
−
θ
)
=
−
tan
(
θ
)
=
tan
(
−
θ
)
{\displaystyle \tan(2\pi -\theta )=-\tan(\theta )=\tan(-\theta )}
csc
(
−
θ
)
=
−
csc
θ
{\displaystyle \csc(-\theta )=-\csc \theta }
csc
(
π
2
−
θ
)
=
sec
θ
{\displaystyle \csc \left({\tfrac {\pi }{2}}-\theta \right)=\sec \theta }
csc
(
π
−
θ
)
=
+
csc
θ
{\displaystyle \csc(\pi -\theta )=+\csc \theta }
csc
(
3
π
2
−
θ
)
=
−
sec
θ
{\displaystyle \csc \left({\tfrac {3\pi }{2}}-\theta \right)=-\sec \theta }
csc
(
2
π
−
θ
)
=
−
csc
(
θ
)
=
csc
(
−
θ
)
{\displaystyle \csc(2\pi -\theta )=-\csc(\theta )=\csc(-\theta )}
sec
(
−
θ
)
=
+
sec
θ
{\displaystyle \sec(-\theta )=+\sec \theta }
sec
(
π
2
−
θ
)
=
csc
θ
{\displaystyle \sec \left({\tfrac {\pi }{2}}-\theta \right)=\csc \theta }
sec
(
π
−
θ
)
=
−
sec
θ
{\displaystyle \sec(\pi -\theta )=-\sec \theta }
sec
(
3
π
2
−
θ
)
=
−
csc
θ
{\displaystyle \sec \left({\tfrac {3\pi }{2}}-\theta \right)=-\csc \theta }
sec
(
2
π
−
θ
)
=
+
sec
(
θ
)
=
sec
(
−
θ
)
{\displaystyle \sec(2\pi -\theta )=+\sec(\theta )=\sec(-\theta )}
cot
(
−
θ
)
=
−
cot
θ
{\displaystyle \cot(-\theta )=-\cot \theta }
cot
(
π
2
−
θ
)
=
tan
θ
{\displaystyle \cot \left({\tfrac {\pi }{2}}-\theta \right)=\tan \theta }
cot
(
π
−
θ
)
=
−
cot
θ
{\displaystyle \cot(\pi -\theta )=-\cot \theta }
cot
(
3
π
2
−
θ
)
=
+
tan
θ
{\displaystyle \cot \left({\tfrac {3\pi }{2}}-\theta \right)=+\tan \theta }
cot
(
2
π
−
θ
)
=
−
cot
(
θ
)
=
cot
(
−
θ
)
{\displaystyle \cot(2\pi -\theta )=-\cot(\theta )=\cot(-\theta )}
Shifts and periodicity [ ]
Transformation of coordinates (
a ,
b ) when shifting the angle
θ
{\displaystyle \theta }
in increments of
π
2
{\displaystyle {\frac {\pi }{2}}}
.
Through shifting the arguments of trigonometric functions by certain angles, changing the sign or applying complementary trigonometric functions can sometimes express particular results more simply. Some examples of shifts are shown below in the table.
A full turn , or
360
∘
,
{\displaystyle 360^{\circ },}
2
π
{\displaystyle 2\pi }
sin , cos , sec , and csc repeat their values and is thus their period. Shifting arguments of any periodic function by any integer multiple of a full period preserves the function value of the unshifted argument.
A half turn , or
180
∘
,
{\displaystyle 180^{\circ },}
π
{\displaystyle \pi }
tan
x
=
sin
x
cos
x
{\displaystyle \tan x={\frac {\sin x}{\cos x}}}
cot
x
=
cos
x
sin
x
,
{\displaystyle \cot x={\frac {\cos x}{\sin x}},}
tan
x
{\displaystyle \tan x}
cot
x
{\displaystyle \cot x}
π
{\displaystyle \pi }
For the functions sin , cos , sec , and csc with period
2
π
,
{\displaystyle 2\pi ,}
2
π
,
{\displaystyle 2\pi ,}
π
,
{\displaystyle \pi ,}
(
2
k
+
1
)
π
,
{\displaystyle (2k+1)\pi ,}
k an arbitrary integer. Any even multiple of
π
{\displaystyle \pi }
A quarter turn , or
90
∘
,
{\displaystyle 90^{\circ },}
π
2
{\displaystyle {\tfrac {\pi }{2}}}
tan
x
{\displaystyle \tan x}
cot
x
{\displaystyle \cot x}
π
{\displaystyle \pi }
180
∘
,
{\displaystyle 180^{\circ },}
(
2
k
+
1
)
π
2
{\displaystyle (2k+1){\tfrac {\pi }{2}}}
For the four other trigonometric functions, a quarter turn also represents a quarter period. A shift by an arbitrary multiple of a quarter period that is not covered by a multiple of half periods can be decomposed in an integer multiple of periods, plus or minus one quarter period. The terms expressing these multiples are
(
4
k
±
1
)
π
2
.
{\displaystyle (4k\pm 1){\tfrac {\pi }{2}}.}
Shifting the arguments of
tan
x
{\displaystyle \tan x}
cot
x
{\displaystyle \cot x}
π
4
{\displaystyle {\tfrac {\pi }{4}}}
Shift by one quarter period
Shift by one half period[10]
Shift by full periods[11]
Period
sin
(
θ
±
π
2
)
=
±
cos
θ
{\displaystyle \sin(\theta \pm {\tfrac {\pi }{2}})=\pm \cos \theta }
sin
(
θ
+
π
)
=
−
sin
θ
{\displaystyle \sin(\theta +\pi )=-\sin \theta }
sin
(
θ
+
k
⋅
2
π
)
=
+
sin
θ
{\displaystyle \sin(\theta +k\cdot 2\pi )=+\sin \theta }
2
π
{\displaystyle 2\pi }
cos
(
θ
±
π
2
)
=
∓
sin
θ
{\displaystyle \cos(\theta \pm {\tfrac {\pi }{2}})=\mp \sin \theta }
cos
(
θ
+
π
)
=
−
cos
θ
{\displaystyle \cos(\theta +\pi )=-\cos \theta }
cos
(
θ
+
k
⋅
2
π
)
=
+
cos
θ
{\displaystyle \cos(\theta +k\cdot 2\pi )=+\cos \theta }
2
π
{\displaystyle 2\pi }
tan
(
θ
±
π
4
)
=
tan
θ
±
1
1
∓
tan
θ
{\displaystyle \tan(\theta \pm {\tfrac {\pi }{4}})={\tfrac {\tan \theta \pm 1}{1\mp \tan \theta }}}
tan
(
θ
+
π
2
)
=
−
cot
θ
{\displaystyle \tan(\theta +{\tfrac {\pi }{2}})=-\cot \theta }
tan
(
θ
+
k
⋅
π
)
=
+
tan
θ
{\displaystyle \tan(\theta +k\cdot \pi )=+\tan \theta }
π
{\displaystyle \pi }
csc
(
θ
±
π
2
)
=
±
sec
θ
{\displaystyle \csc(\theta \pm {\tfrac {\pi }{2}})=\pm \sec \theta }
csc
(
θ
+
π
)
=
−
csc
θ
{\displaystyle \csc(\theta +\pi )=-\csc \theta }
csc
(
θ
+
k
⋅
2
π
)
=
+
csc
θ
{\displaystyle \csc(\theta +k\cdot 2\pi )=+\csc \theta }
2
π
{\displaystyle 2\pi }
sec
(
θ
±
π
2
)
=
∓
csc
θ
{\displaystyle \sec(\theta \pm {\tfrac {\pi }{2}})=\mp \csc \theta }
sec
(
θ
+
π
)
=
−
sec
θ
{\displaystyle \sec(\theta +\pi )=-\sec \theta }
sec
(
θ
+
k
⋅
2
π
)
=
+
sec
θ
{\displaystyle \sec(\theta +k\cdot 2\pi )=+\sec \theta }
2
π
{\displaystyle 2\pi }
cot
(
θ
±
π
4
)
=
cot
θ
±
1
1
∓
cot
θ
{\displaystyle \cot(\theta \pm {\tfrac {\pi }{4}})={\tfrac {\cot \theta \pm 1}{1\mp \cot \theta }}}
cot
(
θ
+
π
2
)
=
−
tan
θ
{\displaystyle \cot(\theta +{\tfrac {\pi }{2}})=-\tan \theta }
cot
(
θ
+
k
⋅
π
)
=
+
cot
θ
{\displaystyle \cot(\theta +k\cdot \pi )=+\cot \theta }
π
{\displaystyle \pi }
Angle sum and difference identities [ ]
Illustration of angle addition formulae for the sine and cosine. Emphasized segment is of unit length.
These are also known as the angle addition and subtraction theorems (or formulae ).
The identities can be derived by combining right triangles such as in the adjacent diagram, or by considering the invariance of the length of a chord on a unit circle given a particular central angle. The most intuitive derivation uses rotation matrices (see below).
Illustration of the angle addition formula for the tangent. Emphasized segments are of unit length.
For acute angles
α
{\displaystyle \alpha }
β
,
{\displaystyle \beta ,}
β
{\displaystyle \beta }
sin
β
{\displaystyle \sin \beta }
cos
β
.
{\displaystyle \cos \beta .}
cos
β
{\displaystyle \cos \beta }
α
{\displaystyle \alpha }
sin
α
{\displaystyle \sin \alpha }
cos
α
,
{\displaystyle \cos \alpha ,}
cos
β
.
{\displaystyle \cos \beta .}
sin
β
{\displaystyle \sin \beta }
α
,
{\displaystyle \alpha ,}
cos
α
cos
β
{\displaystyle \cos \alpha \cos \beta }
sin
α
sin
β
.
{\displaystyle \sin \alpha \sin \beta .}
α
+
β
{\displaystyle \alpha +\beta }
sin
(
α
+
β
)
,
{\displaystyle \sin(\alpha +\beta ),}
cos
(
α
+
β
)
.
{\displaystyle \cos(\alpha +\beta ).}
sin
(
α
+
β
)
=
sin
α
cos
β
+
cos
α
sin
β
cos
(
α
+
β
)
=
cos
α
cos
β
−
sin
α
sin
β
{\displaystyle {\begin{aligned}\sin(\alpha +\beta )&=\sin \alpha \cos \beta +\cos \alpha \sin \beta \\\cos(\alpha +\beta )&=\cos \alpha \cos \beta -\sin \alpha \sin \beta \end{aligned}}}
Relocating one of the named angles yields a variant of the diagram that demonstrates the angle difference formulae for sine and cosine.[12]
cos
α
cos
β
{\displaystyle \cos \alpha \cos \beta }
These identities have applications in, for example, in-phase and quadrature components .
Illustration of the angle addition formula for the cotangent. Top right segment is of unit length.
Sine
sin
(
α
±
β
)
{\displaystyle \sin(\alpha \pm \beta )}
=
{\displaystyle =}
sin
α
cos
β
±
cos
α
sin
β
{\displaystyle \sin \alpha \cos \beta \pm \cos \alpha \sin \beta }
[13] [14]
Cosine
cos
(
α
±
β
)
{\displaystyle \cos(\alpha \pm \beta )}
=
{\displaystyle =}
cos
α
cos
β
∓
sin
α
sin
β
{\displaystyle \cos \alpha \cos \beta \mp \sin \alpha \sin \beta }
[14] [15]
Tangent
tan
(
α
±
β
)
{\displaystyle \tan(\alpha \pm \beta )}
=
{\displaystyle =}
tan
α
±
tan
β
1
∓
tan
α
tan
β
{\displaystyle {\frac {\tan \alpha \pm \tan \beta }{1\mp \tan \alpha \tan \beta }}}
[14] [16]
Cosecant
csc
(
α
±
β
)
{\displaystyle \csc(\alpha \pm \beta )}
=
{\displaystyle =}
sec
α
sec
β
csc
α
csc
β
sec
α
csc
β
±
csc
α
sec
β
{\displaystyle {\frac {\sec \alpha \sec \beta \csc \alpha \csc \beta }{\sec \alpha \csc \beta \pm \csc \alpha \sec \beta }}}
[17]
Secant
sec
(
α
±
β
)
{\displaystyle \sec(\alpha \pm \beta )}
=
{\displaystyle =}
sec
α
sec
β
csc
α
csc
β
csc
α
csc
β
∓
sec
α
sec
β
{\displaystyle {\frac {\sec \alpha \sec \beta \csc \alpha \csc \beta }{\csc \alpha \csc \beta \mp \sec \alpha \sec \beta }}}
[17]
Cotangent
cot
(
α
±
β
)
{\displaystyle \cot(\alpha \pm \beta )}
=
{\displaystyle =}
cot
α
cot
β
∓
1
cot
β
±
cot
α
{\displaystyle {\frac {\cot \alpha \cot \beta \mp 1}{\cot \beta \pm \cot \alpha }}}
[14] [18]
Arcsine
arcsin
x
±
arcsin
y
{\displaystyle \arcsin x\pm \arcsin y}
=
{\displaystyle =}
arcsin
(
x
1
−
y
2
±
y
1
−
x
2
)
{\displaystyle \arcsin \left(x{\sqrt {1-y^{2}}}\pm y{\sqrt {1-x^{2}}}\right)}
[19]
Arccosine
arccos
x
±
arccos
y
{\displaystyle \arccos x\pm \arccos y}
=
{\displaystyle =}
arccos
(
x
y
∓
(
1
−
x
2
)
(
1
−
y
2
)
)
{\displaystyle \arccos \left(xy\mp {\sqrt {\left(1-x^{2}\right)\left(1-y^{2}\right)}}\right)}
[20]
Arctangent
arctan
x
±
arctan
y
{\displaystyle \arctan x\pm \arctan y}
=
{\displaystyle =}
arctan
(
x
±
y
1
∓
x
y
)
{\displaystyle \arctan \left({\frac {x\pm y}{1\mp xy}}\right)}
[21]
Arccotangent
arccot
x
±
arccot
y
{\displaystyle \operatorname {arccot} x\pm \operatorname {arccot} y}
=
{\displaystyle =}
arccot
(
x
y
∓
1
y
±
x
)
{\displaystyle \operatorname {arccot} \left({\frac {xy\mp 1}{y\pm x}}\right)}
Matrix form [ ] The sum and difference formulae for sine and cosine follow from the fact that a rotation of the plane by angle
α
,
{\displaystyle \alpha ,}
β
,
{\displaystyle \beta ,}
α
+
β
.
{\displaystyle \alpha +\beta .}
rotation matrices :
(
cos
α
−
sin
α
sin
α
cos
α
)
(
cos
β
−
sin
β
sin
β
cos
β
)
=
(
cos
α
cos
β
−
sin
α
sin
β
−
cos
α
sin
β
−
sin
α
cos
β
sin
α
cos
β
+
cos
α
sin
β
−
sin
α
sin
β
+
cos
α
cos
β
)
=
(
cos
(
α
+
β
)
−
sin
(
α
+
β
)
sin
(
α
+
β
)
cos
(
α
+
β
)
)
.
{\displaystyle {\begin{aligned}&{}\quad \left({\begin{array}{rr}\cos \alpha &-\sin \alpha \\\sin \alpha &\cos \alpha \end{array}}\right)\left({\begin{array}{rr}\cos \beta &-\sin \beta \\\sin \beta &\cos \beta \end{array}}\right)\\[12pt]&=\left({\begin{array}{rr}\cos \alpha \cos \beta -\sin \alpha \sin \beta &-\cos \alpha \sin \beta -\sin \alpha \cos \beta \\\sin \alpha \cos \beta +\cos \alpha \sin \beta &-\sin \alpha \sin \beta +\cos \alpha \cos \beta \end{array}}\right)\\[12pt]&=\left({\begin{array}{rr}\cos(\alpha +\beta )&-\sin(\alpha +\beta )\\\sin(\alpha +\beta )&\cos(\alpha +\beta )\end{array}}\right).\end{aligned}}}
The matrix inverse for a rotation is the rotation with the negative of the angle
(
cos
α
−
sin
α
sin
α
cos
α
)
−
1
=
(
cos
(
−
α
)
−
sin
(
−
α
)
sin
(
−
α
)
cos
(
−
α
)
)
=
(
cos
α
sin
α
−
sin
α
cos
α
)
,
{\displaystyle \left({\begin{array}{rr}\cos \alpha &-\sin \alpha \\\sin \alpha &\cos \alpha \end{array}}\right)^{-1}=\left({\begin{array}{rr}\cos(-\alpha )&-\sin(-\alpha )\\\sin(-\alpha )&\cos(-\alpha )\end{array}}\right)=\left({\begin{array}{rr}\cos \alpha &\sin \alpha \\-\sin \alpha &\cos \alpha \end{array}}\right)\,,}
which is also the matrix transpose .
These formulae show that these matrices form a representation of the rotation group in the plane (technically, the special orthogonal group SO(2) ), since the composition law is fulfilled and inverses exist. Furthermore, matrix multiplication of the rotation matrix for an angle
α
{\displaystyle \alpha }
α
.
{\displaystyle \alpha .}
Since multiplication by a complex number of unit length rotates the complex plane by the argument of the number, the above multiplication of rotation matrices is equivalent to a multiplication of complex numbers:
(
cos
α
+
i
sin
α
)
(
cos
β
+
i
sin
β
)
=
(
cos
α
cos
β
−
sin
α
sin
β
)
+
i
(
cos
α
sin
β
+
sin
α
cos
β
)
=
cos
(
α
+
β
)
+
i
sin
(
α
+
β
)
.
{\displaystyle {\begin{aligned}(\cos \alpha +i\sin \alpha )(\cos \beta +i\sin \beta )&=(\cos \alpha \cos \beta -\sin \alpha \sin \beta )+i(\cos \alpha \sin \beta +\sin \alpha \cos \beta )\\&=\cos(\alpha +\beta )+i\sin(\alpha +\beta ).\end{aligned}}}
In terms of
Euler's formula , this simply says
e
i
α
e
i
β
=
e
i
(
α
+
β
)
,
{\displaystyle e^{i\alpha }e^{i\beta }=e^{i(\alpha +\beta )},}
showing that
θ
↦
e
i
θ
=
cos
θ
+
i
sin
θ
{\displaystyle \theta \ \mapsto \ e^{i\theta }=\cos \theta +i\sin \theta }
is a one-dimensional complex representation of
S
O
(
2
)
.
{\displaystyle \mathrm {SO} (2).}
Sines and cosines of sums of infinitely many angles [ ] When the series
∑
i
=
1
∞
θ
i
{\textstyle \sum _{i=1}^{\infty }\theta _{i}}
converges absolutely then
sin
(
∑
i
=
1
∞
θ
i
)
=
∑
odd
k
≥
1
(
−
1
)
k
−
1
2
∑
A
⊆
{
1
,
2
,
3
,
…
}
|
A
|
=
k
(
∏
i
∈
A
sin
θ
i
∏
i
∉
A
cos
θ
i
)
{\displaystyle \sin \left(\sum _{i=1}^{\infty }\theta _{i}\right)=\sum _{{\text{odd}}\ k\geq 1}(-1)^{\frac {k-1}{2}}\sum _{\begin{smallmatrix}A\subseteq \{\,1,2,3,\dots \,\}\\\left|A\right|=k\end{smallmatrix}}\left(\prod _{i\in A}\sin \theta _{i}\prod _{i\not \in A}\cos \theta _{i}\right)}
cos
(
∑
i
=
1
∞
θ
i
)
=
∑
even
k
≥
0
(
−
1
)
k
2
∑
A
⊆
{
1
,
2
,
3
,
…
}
|
A
|
=
k
(
∏
i
∈
A
sin
θ
i
∏
i
∉
A
cos
θ
i
)
.
{\displaystyle \cos \left(\sum _{i=1}^{\infty }\theta _{i}\right)=\sum _{{\text{even}}\ k\geq 0}~(-1)^{\frac {k}{2}}~~\sum _{\begin{smallmatrix}A\subseteq \{\,1,2,3,\dots \,\}\\\left|A\right|=k\end{smallmatrix}}\left(\prod _{i\in A}\sin \theta _{i}\prod _{i\not \in A}\cos \theta _{i}\right)\,.}
Because the series
∑
i
=
1
∞
θ
i
{\textstyle \sum _{i=1}^{\infty }\theta _{i}}
lim
i
→
∞
θ
i
=
0
,
{\textstyle \lim _{i\to \infty }\theta _{i}=0,}
lim
i
→
∞
sin
θ
i
=
0
,
{\textstyle \lim _{i\to \infty }\sin \theta _{i}=0,}
lim
i
→
∞
cos
θ
i
=
1.
{\textstyle \lim _{i\to \infty }\cos \theta _{i}=1.}
cofinitely many cosine factors. Terms with infinitely many sine factors would necessarily be equal to zero.
When only finitely many of the angles
θ
i
{\displaystyle \theta _{i}}
Tangents and cotangents of sums [ ] Let
e
k
{\displaystyle e_{k}}
k
=
0
,
1
,
2
,
3
,
…
{\displaystyle k=0,1,2,3,\ldots }
k th-degree elementary symmetric polynomial in the variables
x
i
=
tan
θ
i
{\displaystyle x_{i}=\tan \theta _{i}}
for
i
=
0
,
1
,
2
,
3
,
…
,
{\displaystyle i=0,1,2,3,\ldots ,}
that is,
e
0
=
1
e
1
=
∑
i
x
i
=
∑
i
tan
θ
i
e
2
=
∑
i
<
j
x
i
x
j
=
∑
i
<
j
tan
θ
i
tan
θ
j
e
3
=
∑
i
<
j
<
k
x
i
x
j
x
k
=
∑
i
<
j
<
k
tan
θ
i
tan
θ
j
tan
θ
k
⋮
⋮
{\displaystyle {\begin{aligned}e_{0}&=1\\[6pt]e_{1}&=\sum _{i}x_{i}&&=\sum _{i}\tan \theta _{i}\\[6pt]e_{2}&=\sum _{i<j}x_{i}x_{j}&&=\sum _{i<j}\tan \theta _{i}\tan \theta _{j}\\[6pt]e_{3}&=\sum _{i<j<k}x_{i}x_{j}x_{k}&&=\sum _{i<j<k}\tan \theta _{i}\tan \theta _{j}\tan \theta _{k}\\&{}\ \ \vdots &&{}\ \ \vdots \end{aligned}}}
Then
tan
(
∑
i
θ
i
)
=
sin
(
∑
i
θ
i
)
/
∏
i
cos
θ
i
cos
(
∑
i
θ
i
)
/
∏
i
cos
θ
i
=
∑
odd
k
≥
1
(
−
1
)
k
−
1
2
∑
A
⊆
{
1
,
2
,
3
,
…
}
|
A
|
=
k
∏
i
∈
A
tan
θ
i
∑
even
k
≥
0
(
−
1
)
k
2
∑
A
⊆
{
1
,
2
,
3
,
…
}
|
A
|
=
k
∏
i
∈
A
tan
θ
i
=
e
1
−
e
3
+
e
5
−
⋯
e
0
−
e
2
+
e
4
−
⋯
cot
(
∑
i
θ
i
)
=
e
0
−
e
2
+
e
4
−
⋯
e
1
−
e
3
+
e
5
−
⋯
{\displaystyle {\begin{aligned}\tan \left(\sum _{i}\theta _{i}\right)&={\frac {\sin \left(\sum _{i}\theta _{i}\right)/\prod _{i}\cos \theta _{i}}{\cos \left(\sum _{i}\theta _{i}\right)/\prod _{i}\cos \theta _{i}}}\\&={\frac {\displaystyle \sum _{{\text{odd}}\ k\geq 1}(-1)^{\frac {k-1}{2}}\sum _{\begin{smallmatrix}A\subseteq \{\,1,2,3,\dots \,\}\\\left|A\right|=k\end{smallmatrix}}\prod _{i\in A}\tan \theta _{i}}{\displaystyle \sum _{{\text{even}}\ k\geq 0}~(-1)^{\frac {k}{2}}~~\sum _{\begin{smallmatrix}A\subseteq \{\,1,2,3,\dots \,\}\\\left|A\right|=k\end{smallmatrix}}\prod _{i\in A}\tan \theta _{i}}}={\frac {e_{1}-e_{3}+e_{5}-\cdots }{e_{0}-e_{2}+e_{4}-\cdots }}\\\cot \left(\sum _{i}\theta _{i}\right)&={\frac {e_{0}-e_{2}+e_{4}-\cdots }{e_{1}-e_{3}+e_{5}-\cdots }}\end{aligned}}}
using the sine and cosine sum formulae above.
The number of terms on the right side depends on the number of terms on the left side.
For example:
tan
(
θ
1
+
θ
2
)
=
e
1
e
0
−
e
2
=
x
1
+
x
2
1
−
x
1
x
2
=
tan
θ
1
+
tan
θ
2
1
−
tan
θ
1
tan
θ
2
,
tan
(
θ
1
+
θ
2
+
θ
3
)
=
e
1
−
e
3
e
0
−
e
2
=
(
x
1
+
x
2
+
x
3
)
−
(
x
1
x
2
x
3
)
1
−
(
x
1
x
2
+
x
1
x
3
+
x
2
x
3
)
,
tan
(
θ
1
+
θ
2
+
θ
3
+
θ
4
)
=
e
1
−
e
3
e
0
−
e
2
+
e
4
=
(
x
1
+
x
2
+
x
3
+
x
4
)
−
(
x
1
x
2
x
3
+
x
1
x
2
x
4
+
x
1
x
3
x
4
+
x
2
x
3
x
4
)
1
−
(
x
1
x
2
+
x
1
x
3
+
x
1
x
4
+
x
2
x
3
+
x
2
x
4
+
x
3
x
4
)
+
(
x
1
x
2
x
3
x
4
)
,
{\displaystyle {\begin{aligned}\tan(\theta _{1}+\theta _{2})&={\frac {e_{1}}{e_{0}-e_{2}}}={\frac {x_{1}+x_{2}}{1\ -\ x_{1}x_{2}}}={\frac {\tan \theta _{1}+\tan \theta _{2}}{1\ -\ \tan \theta _{1}\tan \theta _{2}}},\\[8pt]\tan(\theta _{1}+\theta _{2}+\theta _{3})&={\frac {e_{1}-e_{3}}{e_{0}-e_{2}}}={\frac {(x_{1}+x_{2}+x_{3})\ -\ (x_{1}x_{2}x_{3})}{1\ -\ (x_{1}x_{2}+x_{1}x_{3}+x_{2}x_{3})}},\\[8pt]\tan(\theta _{1}+\theta _{2}+\theta _{3}+\theta _{4})&={\frac {e_{1}-e_{3}}{e_{0}-e_{2}+e_{4}}}\\[8pt]&={\frac {(x_{1}+x_{2}+x_{3}+x_{4})\ -\ (x_{1}x_{2}x_{3}+x_{1}x_{2}x_{4}+x_{1}x_{3}x_{4}+x_{2}x_{3}x_{4})}{1\ -\ (x_{1}x_{2}+x_{1}x_{3}+x_{1}x_{4}+x_{2}x_{3}+x_{2}x_{4}+x_{3}x_{4})\ +\ (x_{1}x_{2}x_{3}x_{4})}},\end{aligned}}}
and so on. The case of only finitely many terms can be proved by mathematical induction .[22]
Secants and cosecants of sums [ ]
sec
(
∑
i
θ
i
)
=
∏
i
sec
θ
i
e
0
−
e
2
+
e
4
−
⋯
csc
(
∑
i
θ
i
)
=
∏
i
sec
θ
i
e
1
−
e
3
+
e
5
−
⋯
{\displaystyle {\begin{aligned}\sec \left(\sum _{i}\theta _{i}\right)&={\frac {\prod _{i}\sec \theta _{i}}{e_{0}-e_{2}+e_{4}-\cdots }}\\[8pt]\csc \left(\sum _{i}\theta _{i}\right)&={\frac {\prod _{i}\sec \theta _{i}}{e_{1}-e_{3}+e_{5}-\cdots }}\end{aligned}}}
where
e
k
{\displaystyle e_{k}}
k th-degree elementary symmetric polynomial in the n variables
x
i
=
tan
θ
i
,
{\displaystyle x_{i}=\tan \theta _{i},}
i
=
1
,
…
,
n
,
{\displaystyle i=1,\ldots ,n,}
[23]
For example,
sec
(
α
+
β
+
γ
)
=
sec
α
sec
β
sec
γ
1
−
tan
α
tan
β
−
tan
α
tan
γ
−
tan
β
tan
γ
csc
(
α
+
β
+
γ
)
=
sec
α
sec
β
sec
γ
tan
α
+
tan
β
+
tan
γ
−
tan
α
tan
β
tan
γ
.
{\displaystyle {\begin{aligned}\sec(\alpha +\beta +\gamma )&={\frac {\sec \alpha \sec \beta \sec \gamma }{1-\tan \alpha \tan \beta -\tan \alpha \tan \gamma -\tan \beta \tan \gamma }}\\[8pt]\csc(\alpha +\beta +\gamma )&={\frac {\sec \alpha \sec \beta \sec \gamma }{\tan \alpha +\tan \beta +\tan \gamma -\tan \alpha \tan \beta \tan \gamma }}.\end{aligned}}}
Multiple-angle formulae [ ]
Tn is the n th Chebyshev polynomial
cos
(
n
θ
)
=
T
n
(
cos
θ
)
{\displaystyle \cos(n\theta )=T_{n}(\cos \theta )}
[24]
de Moivre's formula , i is the imaginary unit
cos
(
n
θ
)
+
i
sin
(
n
θ
)
=
(
cos
θ
+
i
sin
θ
)
n
{\displaystyle \cos(n\theta )+i\sin(n\theta )=(\cos \theta +i\sin \theta )^{n}}
[25]
Double-angle, triple-angle, and half-angle formulae [ ] Double-angle formulae [ ] Formulae for twice an angle.[26]
sin
(
2
θ
)
=
2
sin
θ
cos
θ
=
2
tan
θ
1
+
tan
2
θ
{\displaystyle \sin(2\theta )=2\sin \theta \cos \theta ={\frac {2\tan \theta }{1+\tan ^{2}\theta }}}
cos
(
2
θ
)
=
cos
2
θ
−
sin
2
θ
=
2
cos
2
θ
−
1
=
1
−
2
sin
2
θ
=
1
−
tan
2
θ
1
+
tan
2
θ
{\displaystyle \cos(2\theta )=\cos ^{2}\theta -\sin ^{2}\theta =2\cos ^{2}\theta -1=1-2\sin ^{2}\theta ={\frac {1-\tan ^{2}\theta }{1+\tan ^{2}\theta }}}
tan
(
2
θ
)
=
2
tan
θ
1
−
tan
2
θ
{\displaystyle \tan(2\theta )={\frac {2\tan \theta }{1-\tan ^{2}\theta }}}
cot
(
2
θ
)
=
cot
2
θ
−
1
2
cot
θ
{\displaystyle \cot(2\theta )={\frac {\cot ^{2}\theta -1}{2\cot \theta }}}
sec
(
2
θ
)
=
sec
2
θ
2
−
sec
2
θ
{\displaystyle \sec(2\theta )={\frac {\sec ^{2}\theta }{2-\sec ^{2}\theta }}}
csc
(
2
θ
)
=
sec
θ
csc
θ
2
{\displaystyle \csc(2\theta )={\frac {\sec \theta \csc \theta }{2}}}
Triple-angle formulae [ ] Formulae for triple angles.[26]
sin
(
3
θ
)
=
3
sin
θ
−
4
sin
3
θ
=
4
sin
θ
sin
(
π
3
−
θ
)
sin
(
π
3
+
θ
)
{\displaystyle \sin(3\theta )=3\sin \theta -4\sin ^{3}\theta =4\sin \theta \sin \left({\frac {\pi }{3}}-\theta \right)\sin \left({\frac {\pi }{3}}+\theta \right)}
cos
(
3
θ
)
=
4
cos
3
θ
−
3
cos
θ
=
4
cos
θ
cos
(
π
3
−
θ
)
cos
(
π
3
+
θ
)
{\displaystyle \cos(3\theta )=4\cos ^{3}\theta -3\cos \theta =4\cos \theta \cos \left({\frac {\pi }{3}}-\theta \right)\cos \left({\frac {\pi }{3}}+\theta \right)}
tan
(
3
θ
)
=
3
tan
θ
−
tan
3
θ
1
−
3
tan
2
θ
=
tan
θ
tan
(
π
3
−
θ
)
tan
(
π
3
+
θ
)
{\displaystyle \tan(3\theta )={\frac {3\tan \theta -\tan ^{3}\theta }{1-3\tan ^{2}\theta }}=\tan \theta \tan \left({\frac {\pi }{3}}-\theta \right)\tan \left({\frac {\pi }{3}}+\theta \right)}
cot
(
3
θ
)
=
3
cot
θ
−
cot
3
θ
1
−
3
cot
2
θ
{\displaystyle \cot(3\theta )={\frac {3\cot \theta -\cot ^{3}\theta }{1-3\cot ^{2}\theta }}}
sec
(
3
θ
)
=
sec
3
θ
4
−
3
sec
2
θ
{\displaystyle \sec(3\theta )={\frac {\sec ^{3}\theta }{4-3\sec ^{2}\theta }}}
csc
(
3
θ
)
=
csc
3
θ
3
csc
2
θ
−
4
{\displaystyle \csc(3\theta )={\frac {\csc ^{3}\theta }{3\csc ^{2}\theta -4}}}
Half-angle formulae [ ]
sin
θ
2
=
sgn
(
2
π
−
θ
+
4
π
⌊
θ
4
π
⌋
)
1
−
cos
θ
2
sin
2
θ
2
=
1
−
cos
θ
2
cos
θ
2
=
sgn
(
π
+
θ
+
4
π
⌊
π
−
θ
4
π
⌋
)
1
+
cos
θ
2
cos
2
θ
2
=
1
+
cos
θ
2
tan
θ
2
=
csc
θ
−
cot
θ
=
±
1
−
cos
θ
1
+
cos
θ
=
sin
θ
1
+
cos
θ
=
1
−
cos
θ
sin
θ
=
−
1
±
1
+
tan
2
θ
tan
θ
=
tan
θ
1
+
sec
θ
cot
θ
2
=
csc
θ
+
cot
θ
=
±
1
+
cos
θ
1
−
cos
θ
=
sin
θ
1
−
cos
θ
=
1
+
cos
θ
sin
θ
{\displaystyle {\begin{aligned}\sin {\frac {\theta }{2}}&=\operatorname {sgn} \left(2\pi -\theta +4\pi \left\lfloor {\frac {\theta }{4\pi }}\right\rfloor \right){\sqrt {\frac {1-\cos \theta }{2}}}\\[3pt]\sin ^{2}{\frac {\theta }{2}}&={\frac {1-\cos \theta }{2}}\\[3pt]\cos {\frac {\theta }{2}}&=\operatorname {sgn} \left(\pi +\theta +4\pi \left\lfloor {\frac {\pi -\theta }{4\pi }}\right\rfloor \right){\sqrt {\frac {1+\cos \theta }{2}}}\\[3pt]\cos ^{2}{\frac {\theta }{2}}&={\frac {1+\cos \theta }{2}}\\[3pt]\tan {\frac {\theta }{2}}&=\csc \theta -\cot \theta =\pm \,{\sqrt {\frac {1-\cos \theta }{1+\cos \theta }}}={\frac {\sin \theta }{1+\cos \theta }}\\[3pt]&={\frac {1-\cos \theta }{\sin \theta }}={\frac {-1\pm {\sqrt {1+\tan ^{2}\theta }}}{\tan \theta }}={\frac {\tan \theta }{1+\sec {\theta }}}\\[3pt]\cot {\frac {\theta }{2}}&=\csc \theta +\cot \theta =\pm \,{\sqrt {\frac {1+\cos \theta }{1-\cos \theta }}}={\frac {\sin \theta }{1-\cos \theta }}={\frac {1+\cos \theta }{\sin \theta }}\end{aligned}}}
[27] [28]
Also
tan
η
±
θ
2
=
sin
η
±
sin
θ
cos
η
+
cos
θ
tan
(
θ
2
+
π
4
)
=
sec
θ
+
tan
θ
1
−
sin
θ
1
+
sin
θ
=
|
1
−
tan
θ
2
|
|
1
+
tan
θ
2
|
{\displaystyle {\begin{aligned}\tan {\frac {\eta \pm \theta }{2}}&={\frac {\sin \eta \pm \sin \theta }{\cos \eta +\cos \theta }}\\[3pt]\tan \left({\frac {\theta }{2}}+{\frac {\pi }{4}}\right)&=\sec \theta +\tan \theta \\[3pt]{\sqrt {\frac {1-\sin \theta }{1+\sin \theta }}}&={\frac {\left|1-\tan {\frac {\theta }{2}}\right|}{\left|1+\tan {\frac {\theta }{2}}\right|}}\end{aligned}}}
Table [ ] These can be shown by using either the sum and difference identities or the multiple-angle formulae.
Sine
Cosine
Tangent
Cotangent
Double-angle formulae[29] [30]
sin
(
2
θ
)
=
2
sin
θ
cos
θ
=
2
tan
θ
1
+
tan
2
θ
{\displaystyle {\begin{aligned}\sin(2\theta )&=2\sin \theta \cos \theta \ \\&={\frac {2\tan \theta }{1+\tan ^{2}\theta }}\end{aligned}}}
cos
(
2
θ
)
=
cos
2
θ
−
sin
2
θ
=
2
cos
2
θ
−
1
=
1
−
2
sin
2
θ
=
1
−
tan
2
θ
1
+
tan
2
θ
{\displaystyle {\begin{aligned}\cos(2\theta )&=\cos ^{2}\theta -\sin ^{2}\theta \\&=2\cos ^{2}\theta -1\\&=1-2\sin ^{2}\theta \\&={\frac {1-\tan ^{2}\theta }{1+\tan ^{2}\theta }}\end{aligned}}}
tan
(
2
θ
)
=
2
tan
θ
1
−
tan
2
θ
{\displaystyle \tan(2\theta )={\frac {2\tan \theta }{1-\tan ^{2}\theta }}}
cot
(
2
θ
)
=
cot
2
θ
−
1
2
cot
θ
{\displaystyle \cot(2\theta )={\frac {\cot ^{2}\theta -1}{2\cot \theta }}}
Triple-angle formulae[24] [31]
sin
(
3
θ
)
=
−
sin
3
θ
+
3
cos
2
θ
sin
θ
=
−
4
sin
3
θ
+
3
sin
θ
{\displaystyle {\begin{aligned}\sin(3\theta )&=-\sin ^{3}\theta +3\cos ^{2}\theta \sin \theta \\&=-4\sin ^{3}\theta +3\sin \theta \end{aligned}}}
cos
(
3
θ
)
=
cos
3
θ
−
3
sin
2
θ
cos
θ
=
4
cos
3
θ
−
3
cos
θ
{\displaystyle {\begin{aligned}\cos(3\theta )&=\cos ^{3}\theta -3\sin ^{2}\theta \cos \theta \\&=4\cos ^{3}\theta -3\cos \theta \end{aligned}}}
tan
(
3
θ
)
=
3
tan
θ
−
tan
3
θ
1
−
3
tan
2
θ
{\displaystyle \tan(3\theta )={\frac {3\tan \theta -\tan ^{3}\theta }{1-3\tan ^{2}\theta }}}
cot
(
3
θ
)
=
3
cot
θ
−
cot
3
θ
1
−
3
cot
2
θ
{\displaystyle \cot(3\theta )={\frac {3\cot \theta -\cot ^{3}\theta }{1-3\cot ^{2}\theta }}}
Half-angle formulae[27] [28]
sin
θ
2
=
sgn
(
A
)
1
−
cos
θ
2
where
A
=
2
π
−
θ
+
4
π
⌊
θ
4
π
⌋
(
or
sin
2
θ
2
=
1
−
cos
θ
2
)
{\displaystyle {\begin{aligned}&\sin {\frac {\theta }{2}}=\operatorname {sgn}(A)\,{\sqrt {\frac {1-\cos \theta }{2}}}\\\\&{\text{where }}A=2\pi -\theta +4\pi \left\lfloor {\frac {\theta }{4\pi }}\right\rfloor \\\\&\left({\text{or }}\sin ^{2}{\frac {\theta }{2}}={\frac {1-\cos \theta }{2}}\right)\end{aligned}}}
cos
θ
2
=
sgn
(
B
)
1
+
cos
θ
2
where
B
=
π
+
θ
+
4
π
⌊
π
−
θ
4
π
⌋
(
or
cos
2
θ
2
=
1
+
cos
θ
2
)
{\displaystyle {\begin{aligned}&\cos {\frac {\theta }{2}}=\operatorname {sgn}(B)\,{\sqrt {\frac {1+\cos \theta }{2}}}\\\\&{\text{where }}B=\pi +\theta +4\pi \left\lfloor {\frac {\pi -\theta }{4\pi }}\right\rfloor \\\\&\left({\text{or }}\cos ^{2}{\frac {\theta }{2}}={\frac {1+\cos \theta }{2}}\right)\end{aligned}}}
tan
θ
2
=
csc
θ
−
cot
θ
=
±
1
−
cos
θ
1
+
cos
θ
=
sin
θ
1
+
cos
θ
=
1
−
cos
θ
sin
θ
tan
η
+
θ
2
=
sin
η
+
sin
θ
cos
η
+
cos
θ
tan
(
θ
2
+
π
4
)
=
sec
θ
+
tan
θ
1
−
sin
θ
1
+
sin
θ
=
|
1
−
tan
θ
2
|
|
1
+
tan
θ
2
|
tan
θ
2
=
tan
θ
1
+
1
+
tan
2
θ
for
θ
∈
(
−
π
2
,
π
2
)
{\displaystyle {\begin{aligned}\tan {\frac {\theta }{2}}&=\csc \theta -\cot \theta \\&=\pm \,{\sqrt {\frac {1-\cos \theta }{1+\cos \theta }}}\\[3pt]&={\frac {\sin \theta }{1+\cos \theta }}\\[3pt]&={\frac {1-\cos \theta }{\sin \theta }}\\[5pt]\tan {\frac {\eta +\theta }{2}}&={\frac {\sin \eta +\sin \theta }{\cos \eta +\cos \theta }}\\[5pt]\tan \left({\frac {\theta }{2}}+{\frac {\pi }{4}}\right)&=\sec \theta +\tan \theta \\[5pt]{\sqrt {\frac {1-\sin \theta }{1+\sin \theta }}}&={\frac {\left|1-\tan {\frac {\theta }{2}}\right|}{\left|1+\tan {\frac {\theta }{2}}\right|}}\\[5pt]\tan {\frac {\theta }{2}}&={\frac {\tan \theta }{1+{\sqrt {1+\tan ^{2}\theta }}}}\\&{\text{for }}\theta \in \left(-{\tfrac {\pi }{2}},{\tfrac {\pi }{2}}\right)\end{aligned}}}
cot
θ
2
=
csc
θ
+
cot
θ
=
±
1
+
cos
θ
1
−
cos
θ
=
sin
θ
1
−
cos
θ
=
1
+
cos
θ
sin
θ
{\displaystyle {\begin{aligned}\cot {\frac {\theta }{2}}&=\csc \theta +\cot \theta \\&=\pm \,{\sqrt {\frac {1+\cos \theta }{1-\cos \theta }}}\\[3pt]&={\frac {\sin \theta }{1-\cos \theta }}\\[4pt]&={\frac {1+\cos \theta }{\sin \theta }}\end{aligned}}}
The fact that the triple-angle formula for sine and cosine only involves powers of a single function allows one to relate the geometric problem of a compass and straightedge construction of angle trisection to the algebraic problem of solving a cubic equation , which allows one to prove that trisection is in general impossible using the given tools, by field theory .
A formula for computing the trigonometric identities for the one-third angle exists, but it requires finding the zeroes of the cubic equation 4x 3 − 3x + d = 0 , where
x
{\displaystyle x}
d is the known value of the cosine function at the full angle. However, the discriminant of this equation is positive, so this equation has three real roots (of which only one is the solution for the cosine of the one-third angle). None of these solutions is reducible to a real algebraic expression , as they use intermediate complex numbers under the cube roots .
Sine, cosine, and tangent of multiple angles [ ] For specific multiples, these follow from the angle addition formulae, while the general formula was given by 16th-century French mathematician François Viète .[citation needed
sin
(
n
θ
)
=
∑
k
odd
(
−
1
)
k
−
1
2
(
n
k
)
cos
n
−
k
θ
sin
k
θ
=
sin
θ
∑
i
=
0
(
n
+
1
)
/
2
∑
j
=
0
i
(
−
1
)
i
−
j
(
n
2
i
+
1
)
(
i
j
)
cos
n
−
2
(
i
−
j
)
−
1
θ
,
cos
(
n
θ
)
=
∑
k
even
(
−
1
)
k
2
(
n
k
)
cos
n
−
k
θ
sin
k
θ
=
∑
i
=
0
n
/
2
∑
j
=
0
i
(
−
1
)
i
−
j
(
n
2
i
)
(
i
j
)
cos
n
−
2
(
i
−
j
)
θ
,
{\displaystyle {\begin{aligned}\sin(n\theta )&=\sum _{k{\text{ odd}}}(-1)^{\frac {k-1}{2}}{n \choose k}\cos ^{n-k}\theta \sin ^{k}\theta =\sin \theta \sum _{i=0}^{(n+1)/2}\sum _{j=0}^{i}(-1)^{i-j}{n \choose 2i+1}{i \choose j}\cos ^{n-2(i-j)-1}\theta ,\\\cos(n\theta )&=\sum _{k{\text{ even}}}(-1)^{\frac {k}{2}}{n \choose k}\cos ^{n-k}\theta \sin ^{k}\theta =\sum _{i=0}^{n/2}\sum _{j=0}^{i}(-1)^{i-j}{n \choose 2i}{i \choose j}\cos ^{n-2(i-j)}\theta \,,\end{aligned}}}
for nonnegative values of
k
{\displaystyle k}
n
.
{\displaystyle n.}
[citation needed
In each of these two equations, the first parenthesized term is a binomial coefficient , and the final trigonometric function equals one or minus one or zero so that half the entries in each of the sums are removed. The ratio of these formulae gives
tan
(
n
θ
)
=
∑
k
odd
(
−
1
)
k
−
1
2
(
n
k
)
tan
k
θ
∑
k
even
(
−
1
)
k
2
(
n
k
)
tan
k
θ
.
{\displaystyle \tan(n\theta )={\frac {\sum _{k{\text{ odd}}}(-1)^{\frac {k-1}{2}}{n \choose k}\tan ^{k}\theta }{\sum _{k{\text{ even}}}(-1)^{\frac {k}{2}}{n \choose k}\tan ^{k}\theta }}\,.}
[citation needed Chebyshev method [ ] The Chebyshev method is a recursive algorithm for finding the n th multiple angle formula knowing the (n − 1) th and (n − 2) th values.[32]
cos(nx ) can be computed from cos((n − 1)x ) , cos((n − 2)x ) , and cos(x ) with
cos(nx ) = 2 · cos x · cos((n − 1)x ) − cos((n − 2)x ) .This can be proved by adding together the formulae
cos((n − 1)x + x ) = cos((n − 1)x ) cos x − sin((n − 1)x ) sin x cos((n − 1)x − x ) = cos((n − 1)x ) cos x + sin((n − 1)x ) sin x .It follows by induction that cos(nx ) is a polynomial of
cos
x
,
{\displaystyle \cos x,}
Chebyshev polynomials#Trigonometric definition .
Similarly, sin(nx ) can be computed from sin((n − 1)x ) , sin((n − 2)x ) , and cos(x ) with
sin(nx ) = 2 · cos x · sin((n − 1)x ) − sin((n − 2)x ) .This can be proved by adding formulae for sin((n − 1)x + x ) and sin((n − 1)x − x ) .
Serving a purpose similar to that of the Chebyshev method, for the tangent we can write:
tan
(
n
x
)
=
tan
(
(
n
−
1
)
x
)
+
tan
x
1
−
tan
(
(
n
−
1
)
x
)
tan
x
.
{\displaystyle \tan(nx)={\frac {\tan((n-1)x)+\tan x}{1-\tan((n-1)x)\tan x}}\,.}
Tangent of an average [ ]
tan
(
α
+
β
2
)
=
sin
α
+
sin
β
cos
α
+
cos
β
=
−
cos
α
−
cos
β
sin
α
−
sin
β
{\displaystyle \tan \left({\frac {\alpha +\beta }{2}}\right)={\frac {\sin \alpha +\sin \beta }{\cos \alpha +\cos \beta }}=-\,{\frac {\cos \alpha -\cos \beta }{\sin \alpha -\sin \beta }}}
Setting either
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
Viète's infinite product [ ]
cos
θ
2
⋅
cos
θ
4
⋅
cos
θ
8
⋯
=
∏
n
=
1
∞
cos
θ
2
n
=
sin
θ
θ
=
sinc
θ
.
{\displaystyle \cos {\frac {\theta }{2}}\cdot \cos {\frac {\theta }{4}}\cdot \cos {\frac {\theta }{8}}\cdots =\prod _{n=1}^{\infty }\cos {\frac {\theta }{2^{n}}}={\frac {\sin \theta }{\theta }}=\operatorname {sinc} \theta .}
(Refer to Viète's formula and sinc function .)
Power-reduction formulae [ ] Obtained by solving the second and third versions of the cosine double-angle formula.
Sine
Cosine
Other
sin
2
θ
=
1
−
cos
(
2
θ
)
2
{\displaystyle \sin ^{2}\theta ={\frac {1-\cos(2\theta )}{2}}}
cos
2
θ
=
1
+
cos
(
2
θ
)
2
{\displaystyle \cos ^{2}\theta ={\frac {1+\cos(2\theta )}{2}}}
sin
2
θ
cos
2
θ
=
1
−
cos
(
4
θ
)
8
{\displaystyle \sin ^{2}\theta \cos ^{2}\theta ={\frac {1-\cos(4\theta )}{8}}}
sin
3
θ
=
3
sin
θ
−
sin
(
3
θ
)
4
{\displaystyle \sin ^{3}\theta ={\frac {3\sin \theta -\sin(3\theta )}{4}}}
cos
3
θ
=
3
cos
θ
+
cos
(
3
θ
)
4
{\displaystyle \cos ^{3}\theta ={\frac {3\cos \theta +\cos(3\theta )}{4}}}
sin
3
θ
cos
3
θ
=
3
sin
(
2
θ
)
−
sin
(
6
θ
)
32
{\displaystyle \sin ^{3}\theta \cos ^{3}\theta ={\frac {3\sin(2\theta )-\sin(6\theta )}{32}}}
sin
4
θ
=
3
−
4
cos
(
2
θ
)
+
cos
(
4
θ
)
8
{\displaystyle \sin ^{4}\theta ={\frac {3-4\cos(2\theta )+\cos(4\theta )}{8}}}
cos
4
θ
=
3
+
4
cos
(
2
θ
)
+
cos
(
4
θ
)
8
{\displaystyle \cos ^{4}\theta ={\frac {3+4\cos(2\theta )+\cos(4\theta )}{8}}}
sin
4
θ
cos
4
θ
=
3
−
4
cos
(
4
θ
)
+
cos
(
8
θ
)
128
{\displaystyle \sin ^{4}\theta \cos ^{4}\theta ={\frac {3-4\cos(4\theta )+\cos(8\theta )}{128}}}
sin
5
θ
=
10
sin
θ
−
5
sin
(
3
θ
)
+
sin
(
5
θ
)
16
{\displaystyle \sin ^{5}\theta ={\frac {10\sin \theta -5\sin(3\theta )+\sin(5\theta )}{16}}}
cos
5
θ
=
10
cos
θ
+
5
cos
(
3
θ
)
+
cos
(
5
θ
)
16
{\displaystyle \cos ^{5}\theta ={\frac {10\cos \theta +5\cos(3\theta )+\cos(5\theta )}{16}}}
sin
5
θ
cos
5
θ
=
10
sin
(
2
θ
)
−
5
sin
(
6
θ
)
+
sin
(
10
θ
)
512
{\displaystyle \sin ^{5}\theta \cos ^{5}\theta ={\frac {10\sin(2\theta )-5\sin(6\theta )+\sin(10\theta )}{512}}}
and in general terms of powers of
sin
θ
{\displaystyle \sin \theta }
cos
θ
{\displaystyle \cos \theta }
De Moivre's formula , Euler's formula and the binomial theorem [citation needed .
Cosine
Sine
if
n
is odd
{\displaystyle {\text{if }}n{\text{ is odd}}}
cos
n
θ
=
2
2
n
∑
k
=
0
n
−
1
2
(
n
k
)
cos
(
(
n
−
2
k
)
θ
)
{\displaystyle \cos ^{n}\theta ={\frac {2}{2^{n}}}\sum _{k=0}^{\frac {n-1}{2}}{\binom {n}{k}}\cos {{\big (}(n-2k)\theta {\big )}}}
sin
n
θ
=
2
2
n
∑
k
=
0
n
−
1
2
(
−
1
)
(
n
−
1
2
−
k
)
(
n
k
)
sin
(
(
n
−
2
k
)
θ
)
{\displaystyle \sin ^{n}\theta ={\frac {2}{2^{n}}}\sum _{k=0}^{\frac {n-1}{2}}(-1)^{\left({\frac {n-1}{2}}-k\right)}{\binom {n}{k}}\sin {{\big (}(n-2k)\theta {\big )}}}
if
n
is even
{\displaystyle {\text{if }}n{\text{ is even}}}
cos
n
θ
=
1
2
n
(
n
n
2
)
+
2
2
n
∑
k
=
0
n
2
−
1
(
n
k
)
cos
(
(
n
−
2
k
)
θ
)
{\displaystyle \cos ^{n}\theta ={\frac {1}{2^{n}}}{\binom {n}{\frac {n}{2}}}+{\frac {2}{2^{n}}}\sum _{k=0}^{{\frac {n}{2}}-1}{\binom {n}{k}}\cos {{\big (}(n-2k)\theta {\big )}}}
sin
n
θ
=
1
2
n
(
n
n
2
)
+
2
2
n
∑
k
=
0
n
2
−
1
(
−
1
)
(
n
2
−
k
)
(
n
k
)
cos
(
(
n
−
2
k
)
θ
)
{\displaystyle \sin ^{n}\theta ={\frac {1}{2^{n}}}{\binom {n}{\frac {n}{2}}}+{\frac {2}{2^{n}}}\sum _{k=0}^{{\frac {n}{2}}-1}(-1)^{\left({\frac {n}{2}}-k\right)}{\binom {n}{k}}\cos {{\big (}(n-2k)\theta {\big )}}}
Product-to-sum and sum-to-product identities [ ] The product-to-sum identities or prosthaphaeresis formulae can be proven by expanding their right-hand sides using the angle addition theorems . See amplitude modulation for an application of the product-to-sum formulae, and beat (acoustics) and phase detector for applications of the sum-to-product formulae.
Product-to-sum[33]
2
cos
θ
cos
φ
=
cos
(
θ
−
φ
)
+
cos
(
θ
+
φ
)
{\displaystyle 2\cos \theta \cos \varphi ={\cos(\theta -\varphi )+\cos(\theta +\varphi )}}
2
sin
θ
sin
φ
=
cos
(
θ
−
φ
)
−
cos
(
θ
+
φ
)
{\displaystyle 2\sin \theta \sin \varphi ={\cos(\theta -\varphi )-\cos(\theta +\varphi )}}
2
sin
θ
cos
φ
=
sin
(
θ
+
φ
)
+
sin
(
θ
−
φ
)
{\displaystyle 2\sin \theta \cos \varphi ={\sin(\theta +\varphi )+\sin(\theta -\varphi )}}
2
cos
θ
sin
φ
=
sin
(
θ
+
φ
)
−
sin
(
θ
−
φ
)
{\displaystyle 2\cos \theta \sin \varphi ={\sin(\theta +\varphi )-\sin(\theta -\varphi )}}
tan
θ
tan
φ
=
cos
(
θ
−
φ
)
−
cos
(
θ
+
φ
)
cos
(
θ
−
φ
)
+
cos
(
θ
+
φ
)
{\displaystyle \tan \theta \tan \varphi ={\frac {\cos(\theta -\varphi )-\cos(\theta +\varphi )}{\cos(\theta -\varphi )+\cos(\theta +\varphi )}}}
∏
k
=
1
n
cos
θ
k
=
1
2
n
∑
e
∈
S
cos
(
e
1
θ
1
+
⋯
+
e
n
θ
n
)
where
S
=
{
1
,
−
1
}
n
{\displaystyle {\begin{aligned}\prod _{k=1}^{n}\cos \theta _{k}&={\frac {1}{2^{n}}}\sum _{e\in S}\cos(e_{1}\theta _{1}+\cdots +e_{n}\theta _{n})\\[6pt]&{\text{where }}S=\{1,-1\}^{n}\end{aligned}}}
Sum-to-product[34]
sin
θ
±
sin
φ
=
2
sin
(
θ
±
φ
2
)
cos
(
θ
∓
φ
2
)
{\displaystyle \sin \theta \pm \sin \varphi =2\sin \left({\frac {\theta \pm \varphi }{2}}\right)\cos \left({\frac {\theta \mp \varphi }{2}}\right)}
cos
θ
+
cos
φ
=
2
cos
(
θ
+
φ
2
)
cos
(
θ
−
φ
2
)
{\displaystyle \cos \theta +\cos \varphi =2\cos \left({\frac {\theta +\varphi }{2}}\right)\cos \left({\frac {\theta -\varphi }{2}}\right)}
cos
θ
−
cos
φ
=
−
2
sin
(
θ
+
φ
2
)
sin
(
θ
−
φ
2
)
{\displaystyle \cos \theta -\cos \varphi =-2\sin \left({\frac {\theta +\varphi }{2}}\right)\sin \left({\frac {\theta -\varphi }{2}}\right)}
Other related identities [ ]
sec
2
x
+
csc
2
x
=
sec
2
x
csc
2
x
.
{\displaystyle \sec ^{2}x+\csc ^{2}x=\sec ^{2}x\csc ^{2}x.}
[35] If
x
+
y
+
z
=
π
{\displaystyle x+y+z=\pi }
sin
(
2
x
)
+
sin
(
2
y
)
+
sin
(
2
z
)
=
4
sin
x
sin
y
sin
z
.
{\displaystyle \sin(2x)+\sin(2y)+\sin(2z)=4\sin x\sin y\sin z.}
Triple tangent identity: If
x
+
y
+
z
=
π
{\displaystyle x+y+z=\pi }
tan
x
+
tan
y
+
tan
z
=
tan
x
tan
y
tan
z
.
{\displaystyle \tan x+\tan y+\tan z=\tan x\tan y\tan z.}
In particular, the formula holds when
x
,
y
,
and
z
{\displaystyle x,y,{\text{ and }}z}
(If any of
x
,
y
,
z
{\displaystyle x,y,z}
∞
{\displaystyle \infty }
+
∞
{\displaystyle +\infty }
−
∞
{\displaystyle -\infty }
real line , that is approached by
tan
θ
{\displaystyle \tan \theta }
tan
θ
{\displaystyle \tan \theta }
one-point compactification of the real line.) Triple cotangent identity: If
x
+
y
+
z
=
n
π
2
{\displaystyle x+y+z=n{\frac {\pi }{2}}}
n
{\displaystyle n}
cot
x
+
cot
y
+
cot
z
=
cot
x
cot
y
cot
z
.
{\displaystyle \cot x+\cot y+\cot z=\cot x\cot y\cot z.}
Hermite's cotangent identity [ ] Charles Hermite demonstrated the following identity.[36]
a
1
,
…
,
a
n
{\displaystyle a_{1},\ldots ,a_{n}}
complex numbers , no two of which differ by an integer multiple of π . Let
A
n
,
k
=
∏
1
≤
j
≤
n
j
≠
k
cot
(
a
k
−
a
j
)
{\displaystyle A_{n,k}=\prod _{\begin{smallmatrix}1\leq j\leq n\\j\neq k\end{smallmatrix}}\cot(a_{k}-a_{j})}
(in particular,
A
1
,
1
,
{\displaystyle A_{1,1},}
empty product , is 1). Then
cot
(
z
−
a
1
)
⋯
cot
(
z
−
a
n
)
=
cos
n
π
2
+
∑
k
=
1
n
A
n
,
k
cot
(
z
−
a
k
)
.
{\displaystyle \cot(z-a_{1})\cdots \cot(z-a_{n})=\cos {\frac {n\pi }{2}}+\sum _{k=1}^{n}A_{n,k}\cot(z-a_{k}).}
The simplest non-trivial example is the case n = 2
cot
(
z
−
a
1
)
cot
(
z
−
a
2
)
=
−
1
+
cot
(
a
1
−
a
2
)
cot
(
z
−
a
1
)
+
cot
(
a
2
−
a
1
)
cot
(
z
−
a
2
)
.
{\displaystyle \cot(z-a_{1})\cot(z-a_{2})=-1+\cot(a_{1}-a_{2})\cot(z-a_{1})+\cot(a_{2}-a_{1})\cot(z-a_{2}).}
Ptolemy's theorem [ ] Ptolemy's theorem can be expressed in the language of modern trigonometry as:
If w + x + y + z = π
sin
(
w
+
x
)
sin
(
x
+
y
)
=
sin
(
x
+
y
)
sin
(
y
+
z
)
(trivial)
=
sin
(
y
+
z
)
sin
(
z
+
w
)
(trivial)
=
sin
(
z
+
w
)
sin
(
w
+
x
)
(trivial)
=
sin
w
sin
y
+
sin
x
sin
z
.
(significant)
{\displaystyle {\begin{aligned}\sin(w+x)\sin(x+y)&=\sin(x+y)\sin(y+z)&{\text{(trivial)}}\\&=\sin(y+z)\sin(z+w)&{\text{(trivial)}}\\&=\sin(z+w)\sin(w+x)&{\text{(trivial)}}\\&=\sin w\sin y+\sin x\sin z.&{\text{(significant)}}\end{aligned}}}
(The first three equalities are trivial rearrangements; the fourth is the substance of this identity.)
Finite products of trigonometric functions [ ] For coprime integers n , m
∏
k
=
1
n
(
2
a
+
2
cos
(
2
π
k
m
n
+
x
)
)
=
2
(
T
n
(
a
)
+
(
−
1
)
n
+
m
cos
(
n
x
)
)
{\displaystyle \prod _{k=1}^{n}\left(2a+2\cos \left({\frac {2\pi km}{n}}+x\right)\right)=2\left(T_{n}(a)+{(-1)}^{n+m}\cos(nx)\right)}
where Tn is the Chebyshev polynomial .
The following relationship holds for the sine function
∏
k
=
1
n
−
1
sin
(
k
π
n
)
=
n
2
n
−
1
.
{\displaystyle \prod _{k=1}^{n-1}\sin \left({\frac {k\pi }{n}}\right)={\frac {n}{2^{n-1}}}.}
More generally [37]
sin
(
n
x
)
=
2
n
−
1
∏
k
=
0
n
−
1
sin
(
x
+
k
π
n
)
.
{\displaystyle \sin(nx)=2^{n-1}\prod _{k=0}^{n-1}\sin \left(x+{\frac {k\pi }{n}}\right).}
Linear combinations [ ] For some purposes it is important to know that any linear combination of sine waves of the same period or frequency but different phase shifts is also a sine wave with the same period or frequency, but a different phase shift. This is useful in sinusoid data fitting , because the measured or observed data are linearly related to the a and b unknowns of the in-phase and quadrature components basis below, resulting in a simpler Jacobian , compared to that of
c
{\displaystyle c}
φ
{\displaystyle \varphi }
Sine and cosine [ ] The linear combination, or harmonic addition, of sine and cosine waves is equivalent to a single sine wave with a phase shift and scaled amplitude,[38] [39]
a
cos
x
+
b
sin
x
=
c
cos
(
x
+
φ
)
{\displaystyle a\cos x+b\sin x=c\cos(x+\varphi )}
where
c
{\displaystyle c}
φ
{\displaystyle \varphi }
c
=
sgn
(
a
)
a
2
+
b
2
,
φ
=
arctan
(
−
b
a
)
,
{\displaystyle {\begin{aligned}c&=\operatorname {sgn}(a){\sqrt {a^{2}+b^{2}}},\\\varphi &=\operatorname {arctan} \left(-{\frac {b}{a}}\right),\end{aligned}}}
given that
a
≠
0.
{\displaystyle a\neq 0.}
Arbitrary phase shift [ ] More generally, for arbitrary phase shifts, we have
a
sin
(
x
+
θ
a
)
+
b
sin
(
x
+
θ
b
)
=
c
sin
(
x
+
φ
)
{\displaystyle a\sin(x+\theta _{a})+b\sin(x+\theta _{b})=c\sin(x+\varphi )}
where
c
{\displaystyle c}
φ
{\displaystyle \varphi }
c
2
=
a
2
+
b
2
+
2
a
b
cos
(
θ
a
−
θ
b
)
,
tan
φ
=
a
sin
θ
a
+
b
sin
θ
b
a
cos
θ
a
+
b
cos
θ
b
.
{\displaystyle {\begin{aligned}c^{2}&=a^{2}+b^{2}+2ab\cos \left(\theta _{a}-\theta _{b}\right),\\\tan \varphi &={\frac {a\sin \theta _{a}+b\sin \theta _{b}}{a\cos \theta _{a}+b\cos \theta _{b}}}.\end{aligned}}}
More than two sinusoids [ ] The general case reads[39]
∑
i
a
i
sin
(
x
+
θ
i
)
=
a
sin
(
x
+
θ
)
,
{\displaystyle \sum _{i}a_{i}\sin(x+\theta _{i})=a\sin(x+\theta ),}
where
a
2
=
∑
i
,
j
a
i
a
j
cos
(
θ
i
−
θ
j
)
{\displaystyle a^{2}=\sum _{i,j}a_{i}a_{j}\cos(\theta _{i}-\theta _{j})}
and
tan
θ
=
∑
i
a
i
sin
θ
i
∑
i
a
i
cos
θ
i
.
{\displaystyle \tan \theta ={\frac {\sum _{i}a_{i}\sin \theta _{i}}{\sum _{i}a_{i}\cos \theta _{i}}}.}
See also: Phasor addition
Lagrange's trigonometric identities [ ] These identities, named after Joseph Louis Lagrange , are:[40] [41] [42]
∑
n
=
1
N
sin
(
n
θ
)
=
1
2
cot
θ
2
−
cos
(
(
N
+
1
2
)
θ
)
2
sin
(
θ
2
)
∑
n
=
1
N
cos
(
n
θ
)
=
−
1
2
+
sin
(
(
N
+
1
2
)
θ
)
2
sin
(
θ
2
)
{\displaystyle {\begin{aligned}\sum _{n=1}^{N}\sin(n\theta )&={\frac {1}{2}}\cot {\frac {\theta }{2}}-{\frac {\cos \left(\left(N+{\frac {1}{2}}\right)\theta \right)}{2\sin \left({\frac {\theta }{2}}\right)}}\\[5pt]\sum _{n=1}^{N}\cos(n\theta )&=-{\frac {1}{2}}+{\frac {\sin \left(\left(N+{\frac {1}{2}}\right)\theta \right)}{2\sin \left({\frac {\theta }{2}}\right)}}\end{aligned}}}
A related function is the following function of
x
,
{\displaystyle x,}
Dirichlet kernel .
1
+
2
cos
x
+
2
cos
(
2
x
)
+
2
cos
(
3
x
)
+
⋯
+
2
cos
(
n
x
)
=
sin
(
(
n
+
1
2
)
x
)
sin
(
x
2
)
.
{\displaystyle 1+2\cos x+2\cos(2x)+2\cos(3x)+\cdots +2\cos(nx)={\frac {\sin \left(\left(n+{\frac {1}{2}}\right)x\right)}{\sin \left({\frac {x}{2}}\right)}}.}
see proof .
Other sums of trigonometric functions [ ] Sum of sines and cosines with arguments in arithmetic progression:[43]
α
≠
0
,
{\displaystyle \alpha \neq 0,}
sin
φ
+
sin
(
φ
+
α
)
+
sin
(
φ
+
2
α
)
+
⋯
⋯
+
sin
(
φ
+
n
α
)
=
sin
n
+
1
2
α
⋅
sin
(
φ
+
n
2
α
)
sin
1
2
α
and
cos
φ
+
cos
(
φ
+
α
)
+
cos
(
φ
+
2
α
)
+
⋯
⋯
+
cos
(
φ
+
n
α
)
=
sin
n
+
1
2
α
⋅
cos
(
φ
+
n
2
α
)
sin
1
2
α
.
sec
x
±
tan
x
=
tan
(
π
4
±
1
2
x
)
.
{\displaystyle {\begin{aligned}\sin \varphi +\sin(\varphi +\alpha )+\sin(\varphi +2\alpha )&+\cdots \\[8pt]\cdots +\sin(\varphi +n\alpha )&={\frac {\sin {\frac {n+1}{2}}\alpha \cdot \sin \left(\varphi +{\frac {n}{2}}\alpha \right)}{\sin {\frac {1}{2}}\alpha }}\quad {\text{and}}\\[10pt]\cos \varphi +\cos(\varphi +\alpha )+\cos(\varphi +2\alpha )&+\cdots \\[8pt]\cdots +\cos(\varphi +n\alpha )&={\frac {\sin {\frac {n+1}{2}}\alpha \cdot \cos \left(\varphi +{\frac {n}{2}}\alpha \right)}{\sin {\frac {1}{2}}\alpha }}.\\[10pt]\sec x\pm \tan x&=\tan \left({\frac {\pi }{4}}\pm {\frac {1}{2}}x\right).\end{aligned}}}
The above identity is sometimes convenient to know when thinking about the Gudermannian function , which relates the circular and hyperbolic trigonometric functions without resorting to complex numbers .
If
x
,
y
,
and
z
{\displaystyle x,y,{\text{ and }}z}
x
+
y
+
z
=
π
,
{\displaystyle x+y+z=\pi ,}
cot
x
cot
y
+
cot
y
cot
z
+
cot
z
cot
x
=
1.
{\displaystyle \cot x\cot y+\cot y\cot z+\cot z\cot x=1.}
Certain linear fractional transformations [ ] If
f
(
x
)
{\displaystyle f(x)}
linear fractional transformation
f
(
x
)
=
(
cos
α
)
x
−
sin
α
(
sin
α
)
x
+
cos
α
,
{\displaystyle f(x)={\frac {(\cos \alpha )x-\sin \alpha }{(\sin \alpha )x+\cos \alpha }},}
and similarly
g
(
x
)
=
(
cos
β
)
x
−
sin
β
(
sin
β
)
x
+
cos
β
,
{\displaystyle g(x)={\frac {(\cos \beta )x-\sin \beta }{(\sin \beta )x+\cos \beta }},}
then
f
(
g
(
x
)
)
=
g
(
f
(
x
)
)
=
(
cos
(
α
+
β
)
)
x
−
sin
(
α
+
β
)
(
sin
(
α
+
β
)
)
x
+
cos
(
α
+
β
)
.
{\displaystyle f{\big (}g(x){\big )}=g{\big (}f(x){\big )}={\frac {{\big (}\cos(\alpha +\beta ){\big )}x-\sin(\alpha +\beta )}{{\big (}\sin(\alpha +\beta ){\big )}x+\cos(\alpha +\beta )}}.}
More tersely stated, if for all
α
{\displaystyle \alpha }
f
α
{\displaystyle f_{\alpha }}
f
{\displaystyle f}
f
α
∘
f
β
=
f
α
+
β
.
{\displaystyle f_{\alpha }\circ f_{\beta }=f_{\alpha +\beta }.}
If
x
{\displaystyle x}
f
(
x
)
{\displaystyle f(x)}
−
α
.
{\displaystyle -\alpha .}
Inverse trigonometric functions [ ]
arcsin
x
+
arccos
x
=
π
2
arctan
x
+
arccot
x
=
π
2
arctan
x
+
arctan
1
x
=
{
π
2
,
if
x
>
0
−
π
2
,
if
x
<
0
{\displaystyle {\begin{aligned}\arcsin x+\arccos x&={\dfrac {\pi }{2}}\\\arctan x+\operatorname {arccot} x&={\dfrac {\pi }{2}}\\\arctan x+\arctan {\dfrac {1}{x}}&={\begin{cases}{\dfrac {\pi }{2}},&{\text{if }}x>0\\-{\dfrac {\pi }{2}},&{\text{if }}x<0\end{cases}}\end{aligned}}}
arctan
1
x
=
arctan
1
x
+
y
+
arctan
y
x
2
+
x
y
+
1
{\displaystyle \arctan {\frac {1}{x}}=\arctan {\frac {1}{x+y}}+\arctan {\frac {y}{x^{2}+xy+1}}}
[44] Compositions of trig and inverse trig functions [ ]
sin
(
arccos
x
)
=
1
−
x
2
tan
(
arcsin
x
)
=
x
1
−
x
2
sin
(
arctan
x
)
=
x
1
+
x
2
tan
(
arccos
x
)
=
1
−
x
2
x
cos
(
arctan
x
)
=
1
1
+
x
2
cot
(
arcsin
x
)
=
1
−
x
2
x
cos
(
arcsin
x
)
=
1
−
x
2
cot
(
arccos
x
)
=
x
1
−
x
2
{\displaystyle {\begin{aligned}\sin(\arccos x)&={\sqrt {1-x^{2}}}&\tan(\arcsin x)&={\frac {x}{\sqrt {1-x^{2}}}}\\\sin(\arctan x)&={\frac {x}{\sqrt {1+x^{2}}}}&\tan(\arccos x)&={\frac {\sqrt {1-x^{2}}}{x}}\\\cos(\arctan x)&={\frac {1}{\sqrt {1+x^{2}}}}&\cot(\arcsin x)&={\frac {\sqrt {1-x^{2}}}{x}}\\\cos(\arcsin x)&={\sqrt {1-x^{2}}}&\cot(\arccos x)&={\frac {x}{\sqrt {1-x^{2}}}}\end{aligned}}}
Relation to the complex exponential function [ ] With the unit imaginary number
i
{\displaystyle i}
i
2
=
−
1
,
{\displaystyle i^{2}=-1,}
e
i
x
=
cos
x
+
i
sin
x
{\displaystyle e^{ix}=\cos x+i\sin x}
[45] Euler's formula ),
e
−
i
x
=
cos
(
−
x
)
+
i
sin
(
−
x
)
=
cos
x
−
i
sin
x
{\displaystyle e^{-ix}=\cos(-x)+i\sin(-x)=\cos x-i\sin x}
e
i
π
+
1
=
0
{\displaystyle e^{i\pi }+1=0}
Euler's identity ),
e
2
π
i
=
1
{\displaystyle e^{2\pi i}=1}
cos
x
=
e
i
x
+
e
−
i
x
2
{\displaystyle \cos x={\frac {e^{ix}+e^{-ix}}{2}}}
[46]
sin
x
=
e
i
x
−
e
−
i
x
2
i
{\displaystyle \sin x={\frac {e^{ix}-e^{-ix}}{2i}}}
[47]
tan
x
=
sin
x
cos
x
=
e
i
x
−
e
−
i
x
i
(
e
i
x
+
e
−
i
x
)
.
{\displaystyle \tan x={\frac {\sin x}{\cos x}}={\frac {e^{ix}-e^{-ix}}{i({e^{ix}+e^{-ix}})}}\,.}
These formulae are useful for proving many other trigonometric identities. For example, that
e i (θ +φ )e iθ e iφ
cos(θ + φ ) + i sin(θ + φ ) = (cos θ + i sin θ ) (cos φ + i sin φ ) = (cos θ cos φ − sin θ sin φ ) + i (cos θ sin φ + sin θ cos φ ) .That the real part of the left hand side equals the real part of the right hand side is an angle addition formula for cosine. The equality of the imaginary parts gives an angle addition formula for sine.
Infinite product formulae [ ] For applications to special functions , the following infinite product formulae for trigonometric functions are useful:[48] [49]
sin
x
=
x
∏
n
=
1
∞
(
1
−
x
2
π
2
n
2
)
cos
x
=
∏
n
=
1
∞
(
1
−
x
2
π
2
(
n
−
1
2
)
2
)
sinh
x
=
x
∏
n
=
1
∞
(
1
+
x
2
π
2
n
2
)
cosh
x
=
∏
n
=
1
∞
(
1
+
x
2
π
2
(
n
−
1
2
)
2
)
{\displaystyle {\begin{aligned}\sin x&=x\prod _{n=1}^{\infty }\left(1-{\frac {x^{2}}{\pi ^{2}n^{2}}}\right)&\cos x&=\prod _{n=1}^{\infty }\left(1-{\frac {x^{2}}{\pi ^{2}\left(n-{\frac {1}{2}}\right)^{2}}}\right)\\\sinh x&=x\prod _{n=1}^{\infty }\left(1+{\frac {x^{2}}{\pi ^{2}n^{2}}}\right)&\cosh x&=\prod _{n=1}^{\infty }\left(1+{\frac {x^{2}}{\pi ^{2}\left(n-{\frac {1}{2}}\right)^{2}}}\right)\end{aligned}}}
Identities without variables [ ] In terms of the arctangent function we have[44]
arctan
1
2
=
arctan
1
3
+
arctan
1
7
.
{\displaystyle \arctan {\frac {1}{2}}=\arctan {\frac {1}{3}}+\arctan {\frac {1}{7}}.}
The curious identity known as Morrie's law ,
cos
20
∘
⋅
cos
40
∘
⋅
cos
80
∘
=
1
8
,
{\displaystyle \cos 20^{\circ }\cdot \cos 40^{\circ }\cdot \cos 80^{\circ }={\frac {1}{8}},}
is a special case of an identity that contains one variable:
∏
j
=
0
k
−
1
cos
(
2
j
x
)
=
sin
(
2
k
x
)
2
k
sin
x
.
{\displaystyle \prod _{j=0}^{k-1}\cos \left(2^{j}x\right)={\frac {\sin \left(2^{k}x\right)}{2^{k}\sin x}}.}
The same cosine identity in radians is
cos
π
9
cos
2
π
9
cos
4
π
9
=
1
8
.
{\displaystyle \cos {\frac {\pi }{9}}\cos {\frac {2\pi }{9}}\cos {\frac {4\pi }{9}}={\frac {1}{8}}.}
Similarly,
sin
20
∘
⋅
sin
40
∘
⋅
sin
80
∘
=
3
8
{\displaystyle \sin 20^{\circ }\cdot \sin 40^{\circ }\cdot \sin 80^{\circ }={\frac {\sqrt {3}}{8}}}
is a special case of an identity with
x
{\displaystyle x}
sin
x
⋅
sin
(
60
∘
−
x
)
⋅
sin
(
60
∘
+
x
)
=
sin
3
x
4
.
{\displaystyle \sin x\cdot \sin \left(60^{\circ }-x\right)\cdot \sin \left(60^{\circ }+x\right)={\frac {\sin 3x}{4}}.}
For the case
x
{\displaystyle x}
sin
15
∘
⋅
sin
45
∘
⋅
sin
75
∘
=
2
8
,
sin
15
∘
⋅
sin
75
∘
=
1
4
.
{\displaystyle {\begin{aligned}\sin 15^{\circ }\cdot \sin 45^{\circ }\cdot \sin 75^{\circ }&={\frac {\sqrt {2}}{8}},\\\sin 15^{\circ }\cdot \sin 75^{\circ }&={\frac {1}{4}}.\end{aligned}}}
For the case
x
{\displaystyle x}
sin
10
∘
⋅
sin
50
∘
⋅
sin
70
∘
=
1
8
.
{\displaystyle \sin 10^{\circ }\cdot \sin 50^{\circ }\cdot \sin 70^{\circ }={\frac {1}{8}}.}
The same cosine identity is
cos
x
⋅
cos
(
60
∘
−
x
)
⋅
cos
(
60
∘
+
x
)
=
cos
3
x
4
.
{\displaystyle \cos x\cdot \cos \left(60^{\circ }-x\right)\cdot \cos \left(60^{\circ }+x\right)={\frac {\cos 3x}{4}}.}
Similarly,
cos
10
∘
⋅
cos
50
∘
⋅
cos
70
∘
=
3
8
,
cos
15
∘
⋅
cos
45
∘
⋅
cos
75
∘
=
2
8
,
cos
15
∘
⋅
cos
75
∘
=
1
4
.
{\displaystyle {\begin{aligned}\cos 10^{\circ }\cdot \cos 50^{\circ }\cdot \cos 70^{\circ }&={\frac {\sqrt {3}}{8}},\\\cos 15^{\circ }\cdot \cos 45^{\circ }\cdot \cos 75^{\circ }&={\frac {\sqrt {2}}{8}},\\\cos 15^{\circ }\cdot \cos 75^{\circ }&={\frac {1}{4}}.\end{aligned}}}
Similarly,
tan
50
∘
⋅
tan
60
∘
⋅
tan
70
∘
=
tan
80
∘
,
tan
40
∘
⋅
tan
30
∘
⋅
tan
20
∘
=
tan
10
∘
.
{\displaystyle {\begin{aligned}\tan 50^{\circ }\cdot \tan 60^{\circ }\cdot \tan 70^{\circ }&=\tan 80^{\circ },\\\tan 40^{\circ }\cdot \tan 30^{\circ }\cdot \tan 20^{\circ }&=\tan 10^{\circ }.\end{aligned}}}
The following is perhaps not as readily generalized to an identity containing variables (but see explanation below):
cos
24
∘
+
cos
48
∘
+
cos
96
∘
+
cos
168
∘
=
1
2
.
{\displaystyle \cos 24^{\circ }+\cos 48^{\circ }+\cos 96^{\circ }+\cos 168^{\circ }={\frac {1}{2}}.}
Degree measure ceases to be more felicitous than radian measure when we consider this identity with 21 in the denominators:
cos
2
π
21
+
cos
(
2
⋅
2
π
21
)
+
cos
(
4
⋅
2
π
21
)
+
cos
(
5
⋅
2
π
21
)
+
cos
(
8
⋅
2
π
21
)
+
cos
(
10
⋅
2
π
21
)
=
1
2
.
{\displaystyle {\begin{aligned}\cos {\frac {2\pi }{21}}+{}&\cos \left(2\cdot {\frac {2\pi }{21}}\right)+\cos \left(4\cdot {\frac {2\pi }{21}}\right)\\[10pt]{}+{}&\cos \left(5\cdot {\frac {2\pi }{21}}\right)+\cos \left(8\cdot {\frac {2\pi }{21}}\right)+\cos \left(10\cdot {\frac {2\pi }{21}}\right)={\frac {1}{2}}.\end{aligned}}}
The factors 1, 2, 4, 5, 8, 10 may start to make the pattern clear: they are those integers less than 21 / 2 relatively prime to (or have no prime factors in common with) 21. The last several examples are corollaries of a basic fact about the irreducible cyclotomic polynomials : the cosines are the real parts of the zeroes of those polynomials; the sum of the zeroes is the Möbius function evaluated at (in the very last case above) 21; only half of the zeroes are present above. The two identities preceding this last one arise in the same fashion with 21 replaced by 10 and 15, respectively.
Other cosine identities include:[50]
2
cos
π
3
=
1
,
2
cos
π
5
×
2
cos
2
π
5
=
1
,
2
cos
π
7
×
2
cos
2
π
7
×
2
cos
3
π
7
=
1
,
{\displaystyle {\begin{aligned}2\cos {\frac {\pi }{3}}&=1,\\2\cos {\frac {\pi }{5}}\times 2\cos {\frac {2\pi }{5}}&=1,\\2\cos {\frac {\pi }{7}}\times 2\cos {\frac {2\pi }{7}}\times 2\cos {\frac {3\pi }{7}}&=1,\end{aligned}}}
and so forth for all odd numbers, and hence
cos
π
3
+
cos
π
5
×
cos
2
π
5
+
cos
π
7
×
cos
2
π
7
×
cos
3
π
7
+
⋯
=
1.
{\displaystyle \cos {\frac {\pi }{3}}+\cos {\frac {\pi }{5}}\times \cos {\frac {2\pi }{5}}+\cos {\frac {\pi }{7}}\times \cos {\frac {2\pi }{7}}\times \cos {\frac {3\pi }{7}}+\dots =1.}
Many of those curious identities stem from more general facts like the following:
∏
k
=
1
n
−
1
sin
k
π
n
=
n
2
n
−
1
{\displaystyle \prod _{k=1}^{n-1}\sin {\frac {k\pi }{n}}={\frac {n}{2^{n-1}}}}
[51] and
∏
k
=
1
n
−
1
cos
k
π
n
=
sin
π
n
2
2
n
−
1
.
{\displaystyle \prod _{k=1}^{n-1}\cos {\frac {k\pi }{n}}={\frac {\sin {\frac {\pi n}{2}}}{2^{n-1}}}.}
Combining these gives us
∏
k
=
1
n
−
1
tan
k
π
n
=
n
sin
π
n
2
{\displaystyle \prod _{k=1}^{n-1}\tan {\frac {k\pi }{n}}={\frac {n}{\sin {\frac {\pi n}{2}}}}}
If n is an odd number (
n
=
2
m
+
1
{\displaystyle n=2m+1}
∏
k
=
1
m
tan
k
π
2
m
+
1
=
2
m
+
1
{\displaystyle \prod _{k=1}^{m}\tan {\frac {k\pi }{2m+1}}={\sqrt {2m+1}}}
The transfer function of the Butterworth low pass filter can be expressed in terms of polynomial and poles. By setting the frequency as the cutoff frequency, the following identity can be proved:
∏
k
=
1
n
sin
(
2
k
−
1
)
π
4
n
=
∏
k
=
1
n
cos
(
2
k
−
1
)
π
4
n
=
2
2
n
{\displaystyle \prod _{k=1}^{n}\sin {\frac {\left(2k-1\right)\pi }{4n}}=\prod _{k=1}^{n}\cos {\frac {\left(2k-1\right)\pi }{4n}}={\frac {\sqrt {2}}{2^{n}}}}
Computing π [ ] An efficient way to compute π to a large number of digits is based on the following identity without variables, due to Machin . This is known as a Machin-like formula :
π
4
=
4
arctan
1
5
−
arctan
1
239
{\displaystyle {\frac {\pi }{4}}=4\arctan {\frac {1}{5}}-\arctan {\frac {1}{239}}}
or, alternatively, by using an identity of Leonhard Euler :
π
4
=
5
arctan
1
7
+
2
arctan
3
79
{\displaystyle {\frac {\pi }{4}}=5\arctan {\frac {1}{7}}+2\arctan {\frac {3}{79}}}
or by using Pythagorean triples :
π
=
arccos
4
5
+
arccos
5
13
+
arccos
16
65
=
arcsin
3
5
+
arcsin
12
13
+
arcsin
63
65
.
{\displaystyle \pi =\arccos {\frac {4}{5}}+\arccos {\frac {5}{13}}+\arccos {\frac {16}{65}}=\arcsin {\frac {3}{5}}+\arcsin {\frac {12}{13}}+\arcsin {\frac {63}{65}}.}
Others include:
π
4
=
arctan
1
2
+
arctan
1
3
,
{\displaystyle {\frac {\pi }{4}}=\arctan {\frac {1}{2}}+\arctan {\frac {1}{3}},}
[52] [44]
π
=
arctan
1
+
arctan
2
+
arctan
3
,
{\displaystyle \pi =\arctan 1+\arctan 2+\arctan 3,}
[52]
π
4
=
2
arctan
1
3
+
arctan
1
7
.
{\displaystyle {\frac {\pi }{4}}=2\arctan {\frac {1}{3}}+\arctan {\frac {1}{7}}.}
[44] Generally, for numbers t 1 , ..., t n −1θ n n −1k =1t k π /4, 3π /4)t n π /2 − θ n θ n t 1 , ..., t n −1(−1, 1) . In particular, the computed t n t 1 , ..., t n −1
π
2
=
∑
k
=
1
n
arctan
(
t
k
)
π
=
∑
k
=
1
n
sign
(
t
k
)
arccos
(
1
−
t
k
2
1
+
t
k
2
)
π
=
∑
k
=
1
n
arcsin
(
2
t
k
1
+
t
k
2
)
π
=
∑
k
=
1
n
arctan
(
2
t
k
1
−
t
k
2
)
,
{\displaystyle {\begin{aligned}{\frac {\pi }{2}}&=\sum _{k=1}^{n}\arctan(t_{k})\\\pi &=\sum _{k=1}^{n}\operatorname {sign} (t_{k})\arccos \left({\frac {1-t_{k}^{2}}{1+t_{k}^{2}}}\right)\\\pi &=\sum _{k=1}^{n}\arcsin \left({\frac {2t_{k}}{1+t_{k}^{2}}}\right)\\\pi &=\sum _{k=1}^{n}\arctan \left({\frac {2t_{k}}{1-t_{k}^{2}}}\right)\,,\end{aligned}}}
where in all but the first expression, we have used tangent half-angle formulae. The first two formulae work even if one or more of the t k (−1, 1) . Note that if t = p /q (2t , 1 − t 2 , 1 + t 2 ) values in the above formulae are proportional to the Pythagorean triple (2pq , q 2 − p 2 , q 2 + p 2 ) .
For example, for n = 3
π
2
=
arctan
(
a
b
)
+
arctan
(
c
d
)
+
arctan
(
b
d
−
a
c
a
d
+
b
c
)
{\displaystyle {\frac {\pi }{2}}=\arctan \left({\frac {a}{b}}\right)+\arctan \left({\frac {c}{d}}\right)+\arctan \left({\frac {bd-ac}{ad+bc}}\right)}
for any a , b , c , d > 0
A useful mnemonic for certain values of sines and cosines [ ] For certain simple angles, the sines and cosines take the form √n / 2 0 ≤ n ≤ 4 , which makes them easy to remember.
sin
(
0
)
=
sin
(
0
∘
)
=
0
2
=
cos
(
90
∘
)
=
cos
(
π
2
)
sin
(
π
6
)
=
sin
(
30
∘
)
=
1
2
=
cos
(
60
∘
)
=
cos
(
π
3
)
sin
(
π
4
)
=
sin
(
45
∘
)
=
2
2
=
cos
(
45
∘
)
=
cos
(
π
4
)
sin
(
π
3
)
=
sin
(
60
∘
)
=
3
2
=
cos
(
30
∘
)
=
cos
(
π
6
)
sin
(
π
2
)
=
sin
(
90
∘
)
=
4
2
=
cos
(
0
∘
)
=
cos
(
0
)
↑
These
radicands
are
0
,
1
,
2
,
3
,
4.
{\displaystyle {\begin{matrix}\sin \left(0\right)&=&\sin \left(0^{\circ }\right)&=&{\dfrac {\sqrt {0}}{2}}&=&\cos \left(90^{\circ }\right)&=&\cos \left({\dfrac {\pi }{2}}\right)\\[5pt]\sin \left({\dfrac {\pi }{6}}\right)&=&\sin \left(30^{\circ }\right)&=&{\dfrac {\sqrt {1}}{2}}&=&\cos \left(60^{\circ }\right)&=&\cos \left({\dfrac {\pi }{3}}\right)\\[5pt]\sin \left({\dfrac {\pi }{4}}\right)&=&\sin \left(45^{\circ }\right)&=&{\dfrac {\sqrt {2}}{2}}&=&\cos \left(45^{\circ }\right)&=&\cos \left({\dfrac {\pi }{4}}\right)\\[5pt]\sin \left({\dfrac {\pi }{3}}\right)&=&\sin \left(60^{\circ }\right)&=&{\dfrac {\sqrt {3}}{2}}&=&\cos \left(30^{\circ }\right)&=&\cos \left({\dfrac {\pi }{6}}\right)\\[5pt]\sin \left({\dfrac {\pi }{2}}\right)&=&\sin \left(90^{\circ }\right)&=&{\dfrac {\sqrt {4}}{2}}&=&\cos \left(0^{\circ }\right)&=&\cos \left(0\right)\\[5pt]&&&&\uparrow \\&&&&{\text{These}}\\&&&&{\text{radicands}}\\&&&&{\text{are}}\\&&&&0,\,1,\,2,\,3,\,4.\end{matrix}}}
Miscellany [ ] With the golden ratio
φ
{\displaystyle \varphi }
cos
π
5
=
cos
36
∘
=
5
+
1
4
=
φ
2
sin
π
10
=
sin
18
∘
=
5
−
1
4
=
φ
−
1
2
=
1
2
φ
{\displaystyle {\begin{aligned}\cos {\frac {\pi }{5}}&=\cos 36^{\circ }={\frac {{\sqrt {5}}+1}{4}}={\frac {\varphi }{2}}\\[3pt]\sin {\frac {\pi }{10}}&=\sin 18^{\circ }={\frac {{\sqrt {5}}-1}{4}}={\frac {\varphi ^{-1}}{2}}={\frac {1}{2\varphi }}\end{aligned}}}
An identity of Euclid [ ] Euclid showed in Book XIII, Proposition 10 of his Elements
sin
2
18
∘
+
sin
2
30
∘
=
sin
2
36
∘
.
{\displaystyle \sin ^{2}18^{\circ }+\sin ^{2}30^{\circ }=\sin ^{2}36^{\circ }.}
Ptolemy used this proposition to compute some angles in his table of chords .
Composition of trigonometric functions [ ] This identity involves a trigonometric function of a trigonometric function:[53]
cos
(
t
sin
x
)
=
J
0
(
t
)
+
2
∑
k
=
1
∞
J
2
k
(
t
)
cos
(
2
k
x
)
{\displaystyle \cos(t\sin x)=J_{0}(t)+2\sum _{k=1}^{\infty }J_{2k}(t)\cos(2kx)}
sin
(
t
sin
x
)
=
2
∑
k
=
0
∞
J
2
k
+
1
(
t
)
sin
(
(
2
k
+
1
)
x
)
{\displaystyle \sin(t\sin x)=2\sum _{k=0}^{\infty }J_{2k+1}(t)\sin {\big (}(2k+1)x{\big )}}
cos
(
t
cos
x
)
=
J
0
(
t
)
+
2
∑
k
=
1
∞
(
−
1
)
k
J
2
k
(
t
)
cos
(
2
k
x
)
{\displaystyle \cos(t\cos x)=J_{0}(t)+2\sum _{k=1}^{\infty }(-1)^{k}J_{2k}(t)\cos(2kx)}
sin
(
t
cos
x
)
=
2
∑
k
=
0
∞
(
−
1
)
k
J
2
k
+
1
(
t
)
cos
(
(
2
k
+
1
)
x
)
{\displaystyle \sin(t\cos x)=2\sum _{k=0}^{\infty }(-1)^{k}J_{2k+1}(t)\cos {\big (}(2k+1)x{\big )}}
where Ji are Bessel functions .
Calculus [ ] In calculus the relations stated below require angles to be measured in radians ; the relations would become more complicated if angles were measured in another unit such as degrees. If the trigonometric functions are defined in terms of geometry, along with the definitions of arc length and area , their derivatives can be found by verifying two limits. The first is:
lim
x
→
0
sin
x
x
=
1
,
{\displaystyle \lim _{x\rightarrow 0}{\frac {\sin x}{x}}=1,}
verified using the unit circle and squeeze theorem . The second limit is:
lim
x
→
0
1
−
cos
x
x
=
0
,
{\displaystyle \lim _{x\rightarrow 0}{\frac {1-\cos x}{x}}=0,}
verified using the identity tan x / 2 1 − cos x / sin x . Having established these two limits, one can use the limit definition of the derivative and the addition theorems to show that (sin x )′ = cos x and (cos x )′ = −sin x . If the sine and cosine functions are defined by their Taylor series , then the derivatives can be found by differentiating the power series term-by-term.
d
d
x
sin
x
=
cos
x
{\displaystyle {\frac {d}{dx}}\sin x=\cos x}
The rest of the trigonometric functions can be differentiated using the above identities and the rules of differentiation :[54] [55] [56]
d
d
x
sin
x
=
cos
x
,
d
d
x
arcsin
x
=
1
1
−
x
2
d
d
x
cos
x
=
−
sin
x
,
d
d
x
arccos
x
=
−
1
1
−
x
2
d
d
x
tan
x
=
sec
2
x
,
d
d
x
arctan
x
=
1
1
+
x
2
d
d
x
cot
x
=
−
csc
2
x
,
d
d
x
arccot
x
=
−
1
1
+
x
2
d
d
x
sec
x
=
tan
x
sec
x
,
d
d
x
arcsec
x
=
1
|
x
|
x
2
−
1
d
d
x
csc
x
=
−
csc
x
cot
x
,
d
d
x
arccsc
x
=
−
1
|
x
|
x
2
−
1
{\displaystyle {\begin{aligned}{\frac {d}{dx}}\sin x&=\cos x,&{\frac {d}{dx}}\arcsin x&={\frac {1}{\sqrt {1-x^{2}}}}\\\\{\frac {d}{dx}}\cos x&=-\sin x,&{\frac {d}{dx}}\arccos x&={\frac {-1}{\sqrt {1-x^{2}}}}\\\\{\frac {d}{dx}}\tan x&=\sec ^{2}x,&{\frac {d}{dx}}\arctan x&={\frac {1}{1+x^{2}}}\\\\{\frac {d}{dx}}\cot x&=-\csc ^{2}x,&{\frac {d}{dx}}\operatorname {arccot} x&={\frac {-1}{1+x^{2}}}\\\\{\frac {d}{dx}}\sec x&=\tan x\sec x,&{\frac {d}{dx}}\operatorname {arcsec} x&={\frac {1}{|x|{\sqrt {x^{2}-1}}}}\\\\{\frac {d}{dx}}\csc x&=-\csc x\cot x,&{\frac {d}{dx}}\operatorname {arccsc} x&={\frac {-1}{|x|{\sqrt {x^{2}-1}}}}\end{aligned}}}
The integral identities can be found in List of integrals of trigonometric functions . Some generic forms are listed below.
∫
d
u
a
2
−
u
2
=
sin
−
1
(
u
a
)
+
C
∫
d
u
a
2
+
u
2
=
1
a
tan
−
1
(
u
a
)
+
C
∫
d
u
u
u
2
−
a
2
=
1
a
sec
−
1
|
u
a
|
+
C
{\displaystyle {\begin{aligned}\int {\frac {du}{\sqrt {a^{2}-u^{2}}}}&=\sin ^{-1}\left({\frac {u}{a}}\right)+C\\[3pt]\int {\frac {du}{a^{2}+u^{2}}}&={\frac {1}{a}}\tan ^{-1}\left({\frac {u}{a}}\right)+C\\[3pt]\int {\frac {du}{u{\sqrt {u^{2}-a^{2}}}}}&={\frac {1}{a}}\sec ^{-1}\left|{\frac {u}{a}}\right|+C\end{aligned}}}
Implications [ ] The fact that the differentiation of trigonometric functions (sine and cosine) results in linear combinations of the same two functions is of fundamental importance to many fields of mathematics, including differential equations and Fourier transforms .
Some differential equations satisfied by the sine function [ ] Let
i
=
−
1
{\displaystyle i={\sqrt {-1}}}
odd positive integer n ,
∑
k
=
0
n
(
n
k
)
(
d
d
x
−
sin
x
)
∘
(
d
d
x
−
sin
x
+
i
)
∘
⋯
⋯
∘
(
d
d
x
−
sin
x
+
(
k
−
1
)
i
)
(
sin
x
)
n
−
k
=
0.
{\displaystyle {\begin{aligned}\sum _{k=0}^{n}{\binom {n}{k}}\left({\frac {d}{dx}}-\sin x\right)&\circ \left({\frac {d}{dx}}-\sin x+i\right)\circ \cdots \\\cdots &\circ \left({\frac {d}{dx}}-\sin x+(k-1)i\right)(\sin x)^{n-k}=0.\end{aligned}}}
(When k = 0, then the number of differential operators being composed is 0, so the corresponding term in the sum above is just (sin x )n .) This identity was discovered as a by-product of research in medical imaging .[57]
Exponential definitions [ ]
Function
Inverse function[58]
sin
θ
=
e
i
θ
−
e
−
i
θ
2
i
{\displaystyle \sin \theta ={\frac {e^{i\theta }-e^{-i\theta }}{2i}}}
arcsin
x
=
−
i
ln
(
i
x
+
1
−
x
2
)
{\displaystyle \arcsin x=-i\,\ln \left(ix+{\sqrt {1-x^{2}}}\right)}
cos
θ
=
e
i
θ
+
e
−
i
θ
2
{\displaystyle \cos \theta ={\frac {e^{i\theta }+e^{-i\theta }}{2}}}
arccos
x
=
−
i
ln
(
x
+
x
2
−
1
)
{\displaystyle \arccos x=-i\,\ln \left(x+\,{\sqrt {x^{2}-1}}\right)}
tan
θ
=
−
i
e
i
θ
−
e
−
i
θ
e
i
θ
+
e
−
i
θ
{\displaystyle \tan \theta =-i\,{\frac {e^{i\theta }-e^{-i\theta }}{e^{i\theta }+e^{-i\theta }}}}
arctan
x
=
i
2
ln
(
i
+
x
i
−
x
)
{\displaystyle \arctan x={\frac {i}{2}}\ln \left({\frac {i+x}{i-x}}\right)}
csc
θ
=
2
i
e
i
θ
−
e
−
i
θ
{\displaystyle \csc \theta ={\frac {2i}{e^{i\theta }-e^{-i\theta }}}}
arccsc
x
=
−
i
ln
(
i
x
+
1
−
1
x
2
)
{\displaystyle \operatorname {arccsc} x=-i\,\ln \left({\frac {i}{x}}+{\sqrt {1-{\frac {1}{x^{2}}}}}\right)}
sec
θ
=
2
e
i
θ
+
e
−
i
θ
{\displaystyle \sec \theta ={\frac {2}{e^{i\theta }+e^{-i\theta }}}}
arcsec
x
=
−
i
ln
(
1
x
+
i
1
−
1
x
2
)
{\displaystyle \operatorname {arcsec} x=-i\,\ln \left({\frac {1}{x}}+i{\sqrt {1-{\frac {1}{x^{2}}}}}\right)}
cot
θ
=
i
e
i
θ
+
e
−
i
θ
e
i
θ
−
e
−
i
θ
{\displaystyle \cot \theta =i\,{\frac {e^{i\theta }+e^{-i\theta }}{e^{i\theta }-e^{-i\theta }}}}
arccot
x
=
i
2
ln
(
x
−
i
x
+
i
)
{\displaystyle \operatorname {arccot} x={\frac {i}{2}}\ln \left({\frac {x-i}{x+i}}\right)}
cis
θ
=
e
i
θ
{\displaystyle \operatorname {cis} \theta =e^{i\theta }}
arccis
x
=
−
i
ln
x
{\displaystyle \operatorname {arccis} x=-i\ln x}
Further "conditional" identities for the case α + β + γ = 180° [ ] The following formulae apply to arbitrary plane triangles and follow from
α
+
β
+
γ
=
180
∘
,
{\displaystyle \alpha +\beta +\gamma =180^{\circ },}
tan
α
+
tan
β
+
tan
γ
=
tan
α
tan
β
tan
γ
1
=
cot
β
cot
γ
+
cot
γ
cot
α
+
cot
α
cot
β
cot
(
α
2
)
+
cot
(
β
2
)
+
cot
(
γ
2
)
=
cot
(
α
2
)
cot
(
β
2
)
cot
(
γ
2
)
1
=
tan
(
β
2
)
tan
(
γ
2
)
+
tan
(
γ
2
)
tan
(
α
2
)
+
tan
(
α
2
)
tan
(
β
2
)
sin
α
+
sin
β
+
sin
γ
=
4
cos
(
α
2
)
cos
(
β
2
)
cos
(
γ
2
)
−
sin
α
+
sin
β
+
sin
γ
=
4
cos
(
α
2
)
sin
(
β
2
)
sin
(
γ
2
)
cos
α
+
cos
β
+
cos
γ
=
4
sin
(
α
2
)
sin
(
β
2
)
sin
(
γ
2
)
+
1
−
cos
α
+
cos
β
+
cos
γ
=
4
sin
(
α
2
)
cos
(
β
2
)
cos
(
γ
2
)
−
1
sin
(
2
α
)
+
sin
(
2
β
)
+
sin
(
2
γ
)
=
4
sin
α
sin
β
sin
γ
−
sin
(
2
α
)
+
sin
(
2
β
)
+
sin
(
2
γ
)
=
4
sin
α
cos
β
cos
γ
cos
(
2
α
)
+
cos
(
2
β
)
+
cos
(
2
γ
)
=
−
4
cos
α
cos
β
cos
γ
−
1
−
cos
(
2
α
)
+
cos
(
2
β
)
+
cos
(
2
γ
)
=
−
4
cos
α
sin
β
sin
γ
+
1
sin
2
α
+
sin
2
β
+
sin
2
γ
=
2
cos
α
cos
β
cos
γ
+
2
−
sin
2
α
+
sin
2
β
+
sin
2
γ
=
2
cos
α
sin
β
sin
γ
cos
2
α
+
cos
2
β
+
cos
2
γ
=
−
2
cos
α
cos
β
cos
γ
+
1
−
cos
2
α
+
cos
2
β
+
cos
2
γ
=
−
2
cos
α
sin
β
sin
γ
+
1
−
sin
2
(
2
α
)
+
sin
2
(
2
β
)
+
sin
2
(
2
γ
)
=
−
2
cos
(
2
α
)
sin
(
2
β
)
sin
(
2
γ
)
−
cos
2
(
2
α
)
+
cos
2
(
2
β
)
+
cos
2
(
2
γ
)
=
2
cos
(
2
α
)
sin
(
2
β
)
sin
(
2
γ
)
+
1
1
=
sin
2
(
α
2
)
+
sin
2
(
β
2
)
+
sin
2
(
γ
2
)
+
2
sin
(
α
2
)
sin
(
β
2
)
sin
(
γ
2
)
{\displaystyle {\begin{aligned}\tan \alpha +\tan \beta +\tan \gamma &=\tan \alpha \tan \beta \tan \gamma \\1&=\cot \beta \cot \gamma +\cot \gamma \cot \alpha +\cot \alpha \cot \beta \\\cot \left({\frac {\alpha }{2}}\right)+\cot \left({\frac {\beta }{2}}\right)+\cot \left({\frac {\gamma }{2}}\right)&=\cot \left({\frac {\alpha }{2}}\right)\cot \left({\frac {\beta }{2}}\right)\cot \left({\frac {\gamma }{2}}\right)\\1&=\tan \left({\frac {\beta }{2}}\right)\tan \left({\frac {\gamma }{2}}\right)+\tan \left({\frac {\gamma }{2}}\right)\tan \left({\frac {\alpha }{2}}\right)+\tan \left({\frac {\alpha }{2}}\right)\tan \left({\frac {\beta }{2}}\right)\\\sin \alpha +\sin \beta +\sin \gamma &=4\cos \left({\frac {\alpha }{2}}\right)\cos \left({\frac {\beta }{2}}\right)\cos \left({\frac {\gamma }{2}}\right)\\-\sin \alpha +\sin \beta +\sin \gamma &=4\cos \left({\frac {\alpha }{2}}\right)\sin \left({\frac {\beta }{2}}\right)\sin \left({\frac {\gamma }{2}}\right)\\\cos \alpha +\cos \beta +\cos \gamma &=4\sin \left({\frac {\alpha }{2}}\right)\sin \left({\frac {\beta }{2}}\right)\sin \left({\frac {\gamma }{2}}\right)+1\\-\cos \alpha +\cos \beta +\cos \gamma &=4\sin \left({\frac {\alpha }{2}}\right)\cos \left({\frac {\beta }{2}}\right)\cos \left({\frac {\gamma }{2}}\right)-1\\\sin(2\alpha )+\sin(2\beta )+\sin(2\gamma )&=4\sin \alpha \sin \beta \sin \gamma \\-\sin(2\alpha )+\sin(2\beta )+\sin(2\gamma )&=4\sin \alpha \cos \beta \cos \gamma \\\cos(2\alpha )+\cos(2\beta )+\cos(2\gamma )&=-4\cos \alpha \cos \beta \cos \gamma -1\\-\cos(2\alpha )+\cos(2\beta )+\cos(2\gamma )&=-4\cos \alpha \sin \beta \sin \gamma +1\\\sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma &=2\cos \alpha \cos \beta \cos \gamma +2\\-\sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma &=2\cos \alpha \sin \beta \sin \gamma \\\cos ^{2}\alpha +\cos ^{2}\beta +\cos ^{2}\gamma &=-2\cos \alpha \cos \beta \cos \gamma +1\\-\cos ^{2}\alpha +\cos ^{2}\beta +\cos ^{2}\gamma &=-2\cos \alpha \sin \beta \sin \gamma +1\\-\sin ^{2}(2\alpha )+\sin ^{2}(2\beta )+\sin ^{2}(2\gamma )&=-2\cos(2\alpha )\sin(2\beta )\sin(2\gamma )\\-\cos ^{2}(2\alpha )+\cos ^{2}(2\beta )+\cos ^{2}(2\gamma )&=2\cos(2\alpha )\,\sin(2\beta )\,\sin(2\gamma )+1\\1&=\sin ^{2}\left({\frac {\alpha }{2}}\right)+\sin ^{2}\left({\frac {\beta }{2}}\right)+\sin ^{2}\left({\frac {\gamma }{2}}\right)+2\sin \left({\frac {\alpha }{2}}\right)\,\sin \left({\frac {\beta }{2}}\right)\,\sin \left({\frac {\gamma }{2}}\right)\end{aligned}}}
Miscellaneous [ ] Dirichlet kernel [ ] The Dirichlet kernel Dn (x )
1
+
2
cos
x
+
2
cos
(
2
x
)
+
2
cos
(
3
x
)
+
⋯
+
2
cos
(
n
x
)
=
sin
(
(
n
+
1
2
)
x
)
sin
(
1
2
x
)
.
{\displaystyle 1+2\cos x+2\cos(2x)+2\cos(3x)+\cdots +2\cos(nx)={\frac {\sin \left(\left(n+{\frac {1}{2}}\right)x\right)}{\sin \left({\frac {1}{2}}x\right)}}.}
The convolution of any integrable function of period
2
π
{\displaystyle 2\pi }
n
{\displaystyle n}
measure or generalized function .
Tangent half-angle substitution [ ] Main article: Tangent half-angle substitution
If we set
t
=
tan
x
2
,
{\displaystyle t=\tan {\frac {x}{2}},}
then[59]
sin
x
=
2
t
1
+
t
2
;
cos
x
=
1
−
t
2
1
+
t
2
;
e
i
x
=
1
+
i
t
1
−
i
t
{\displaystyle \sin x={\frac {2t}{1+t^{2}}};\qquad \cos x={\frac {1-t^{2}}{1+t^{2}}};\qquad e^{ix}={\frac {1+it}{1-it}}}
where
e
i
x
=
cos
x
+
i
sin
x
,
{\displaystyle e^{ix}=\cos x+i\sin x,}
cis x .
When this substitution of
t
{\displaystyle t}
tan x / 2 is used in calculus , it follows that
sin
x
{\displaystyle \sin x}
2t / 1 + t 2
cos
x
{\displaystyle \cos x}
1 − t 2 / 1 + t 2 dx is replaced by 2 dt / 1 + t 2
sin
x
{\displaystyle \sin x}
cos
x
{\displaystyle \cos x}
t
{\displaystyle t}
antiderivatives .
See also [ ] Notes [ ]
^ Heng, Cheng and Talbert, "Additional Mathematics" , page 228
^ Schaumberger, N. (1974). "A Classroom Theorem on Trigonometric Irrationalities". Two-Year College Math. J . 5 (1): 73–76. doi :10.2307/3026991 . JSTOR 3026991 . ^ Garibaldi, Skip (June 2008). "Somewhat More than Governors Need to Know about Trigonometry" . Mathematics Magazine . 81 (3): 191–200. doi :10.1080/0025570X.2008.11953548 . ISSN 0025-570X . ^ Weisstein, Eric W. "Niven's Theorem" . MathWorld ^ Abramowitz and Stegun, p. 73, 4.3.45
^ Abramowitz and Stegun, p. 78, 4.3.147
^ Nielsen (1966 , pp. xxiii–xxiv)^ Selby 1970 , p. 188^ Abramowitz and Stegun, p. 72, 4.3.13–15
^ Abramowitz and Stegun, p. 72, 4.3.9
^ Abramowitz and Stegun, p. 72, 4.3.7–8
^ The Trigonographer (28 September 2015). "Angle Sum and Difference for Sine and Cosine" . Trigonography.com . Retrieved 28 May 2017 . ^ Abramowitz and Stegun, p. 72, 4.3.16
^ Jump up to: a b c d Weisstein, Eric W. "Trigonometric Addition Formulas" . MathWorld ^ Abramowitz and Stegun, p. 72, 4.3.17
^ Abramowitz and Stegun, p. 72, 4.3.18
^ Jump up to: a b "Angle Sum and Difference Identities" . www.milefoot.com . Retrieved 2019-10-12 .^ Abramowitz and Stegun, p. 72, 4.3.19
^ Abramowitz and Stegun, p. 80, 4.4.32
^ Abramowitz and Stegun, p. 80, 4.4.33
^ Abramowitz and Stegun, p. 80, 4.4.34
^ Bronstein, Manuel (1989). "Simplification of real elementary functions". In Gonnet, G. H. (ed.). Proceedings of the ACM-SIGSAM 1989 International Symposium on Symbolic and Algebraic Computation . ISSAC '89 (Portland US-OR, 1989-07). New York: ACM . pp. 207–211. doi :10.1145/74540.74566 . ISBN 0-89791-325-6 ^ Michael Hardy (August–September 2016). "On Tangents and Secants of Infinite Sums" . American Mathematical Monthly . 123 (7): 701–703. doi :10.4169/amer.math.monthly.123.7.701 . ^ Jump up to: a b Weisstein, Eric W. "Multiple-Angle Formulas" . MathWorld ^ Abramowitz and Stegun, p. 74, 4.3.48
^ Jump up to: a b Selby 1970 , pg. 190^ Jump up to: a b Abramowitz and Stegun, p. 72, 4.3.20–22
^ Jump up to: a b Weisstein, Eric W. "Half-Angle Formulas" . MathWorld ^ Abramowitz and Stegun, p. 72, 4.3.24–26
^ Weisstein, Eric W. "Double-Angle Formulas" . MathWorld ^ Abramowitz and Stegun, p. 72, 4.3.27–28
^ Ward, Ken. "Multiple angles recursive formula" . Ken Ward's Mathematics Pages . ^ Abramowitz and Stegun, p. 72, 4.3.31–33
^ Abramowitz and Stegun, p. 72, 4.3.34–39
^ Nelson, Roger. "Mathematics Without Words", The College Mathematics Journal 33(2), March 2002, p. 130.
^ Johnson, Warren P. (Apr 2010). "Trigonometric Identities à la Hermite". American Mathematical Monthly 117 (4): 311–327. doi :10.4169/000298910x480784 . ^ "Product Identity Multiple Angle" .^ Apostol, T.M. (1967) Calculus. 2nd edition. New York, NY, Wiley. Pp 334-335.
^ Jump up to: a b Weisstein, Eric W. "Harmonic Addition Theorem" . MathWorld ^ Ortiz Muñiz, Eddie (Feb 1953). "A Method for Deriving Various Formulas in Electrostatics and Electromagnetism Using Lagrange's Trigonometric Identities". American Journal of Physics . 21 (2): 140. Bibcode :1953AmJPh..21..140M . doi :10.1119/1.1933371 . ^ Agarwal, Ravi P.; O'Regan, Donal (2008). Ordinary and Partial Differential Equations: With Special Functions, Fourier Series, and Boundary Value Problems ISBN 9780387791463 Extract of page 185 ^ Jeffrey, Alan; Dai, Hui-hui (2008). "Section 2.4.1.6". Handbook of Mathematical Formulas and Integrals (4th ed.). Academic Press. ISBN 978-0-12-374288-9 ^ Knapp, Michael P. "Sines and Cosines of Angles in Arithmetic Progression" (PDF) . ^ Jump up to: a b c d Wu, Rex H. "Proof Without Words: Euler's Arctangent Identity", Mathematics Magazine 77(3), June 2004, p. 189.
^ Abramowitz and Stegun, p. 74,
4.3.47
^ Abramowitz and Stegun, p. 71,
4.3.2
^ Abramowitz and Stegun, p. 71,
4.3.1
^ Abramowitz and Stegun, p. 75, 4.3.89–90
^ Abramowitz and Stegun, p. 85, 4.5.68–69
^ Humble, Steve (Nov 2004). "Grandma's identity". Mathematical Gazette . 88 : 524–525. doi :10.1017/s0025557200176223 . ^ Weisstein, Eric W. "Sine" . MathWorld ^ Jump up to: a b Harris, Edward M. "Sums of Arctangents", in Roger B. Nelson, Proofs Without Words (1993, Mathematical Association of America), p. 39.
^ Milton Abramowitz and Irene Stegun, Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables , Dover Publications , New York, 1972, formulae 9.1.42–9.1.45^ Abramowitz and Stegun, p. 77, 4.3.105–110
^ Abramowitz and Stegun, p. 82, 4.4.52–57
^ Finney, Ross (2003). Calculus : Graphical, Numerical, Algebraic 159–161 . ISBN 0-13-063131-0 ^ Kuchment, Peter; Lvin, Sergey (Aug 2013). "Identities for sin x that Came from Medical Imaging". American Mathematical Monthly 120 (7): 609–621. arXiv :1110.6109 doi :10.4169/amer.math.monthly.120.07.609 . ^ Abramowitz and Stegun, p. 80, 4.4.26–31
^ Abramowitz and Stegun, p. 72, 4.3.23
References [ ] Abramowitz, Milton ; Stegun, Irene A. , eds. (1972). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables Dover Publications . ISBN 978-0-486-61272-0 Nielsen, Kaj L. (1966), Logarithmic and Trigonometric Tables to Five Places (2nd ed.), New York: Barnes & Noble , LCCN 61-9103 Selby, Samuel M., ed. (1970), Standard Mathematical Tables (18th ed.), The Chemical Rubber Co. External links [ ] 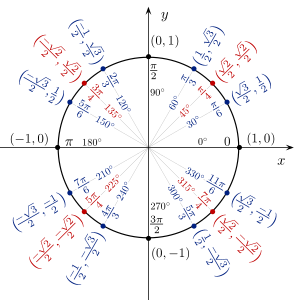





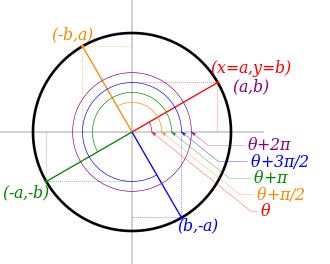
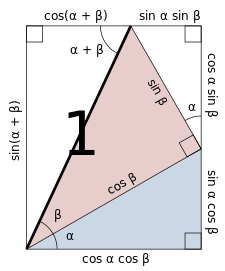


















































































































![[-1, 1]](https://wikimedia.org/api/rest_v1/media/math/render/svg/51e3b7f14a6f70e614728c583409a0b9a8b9de01)

![{\displaystyle \left[-{\frac {\pi }{2}},{\frac {\pi }{2}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/54381f086ac9ffe8306d413f813abcb616e95dee)

![[0,\pi ]](https://wikimedia.org/api/rest_v1/media/math/render/svg/3e2a912eda6ef1afe46a81b518fe9da64a832751)







![{\displaystyle (-\infty ,-1]\cup [1,\infty )}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7e3448e2de68557e598967fb8b1f8900260c4a64)

![{\displaystyle \left[0,{\frac {\pi }{2}}\right)\cup \left({\frac {\pi }{2}},\pi \right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/87897be4e87384b3bf3bae73022748792bc19644)

![{\displaystyle \left[-{\frac {\pi }{2}},0\right)\cup \left(0,{\frac {\pi }{2}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7514aa3d834058e5180511ffa1b9c445fc3913ce)
















![{\displaystyle {\begin{alignedat}{9}{\frac {\pi }{2}}~&=~\arcsin(x)&&+\arccos(x)~&&=~\arctan(r)&&+\operatorname {arccot}(r)~&&=~\operatorname {arcsec}(s)&&+\operatorname {arccsc}(s)\\[0.4ex]\pi ~&=~\arccos(x)&&+\arccos(-x)~&&=~\operatorname {arccot}(r)&&+\operatorname {arccot}(-r)~&&=~\operatorname {arcsec}(s)&&+\operatorname {arcsec}(-s)\\[0.4ex]0~&=~\arcsin(x)&&+\arcsin(-x)~&&=~\arctan(r)&&+\arctan(-r)~&&=~\operatorname {arccsc}(s)&&+\operatorname {arccsc}(-s)\\[1.0ex]\end{alignedat}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/839db041f421b4af5890106450e2b4d3e9e183d4)







































































































































































































































































![{\displaystyle {\begin{aligned}&{}\quad \left({\begin{array}{rr}\cos \alpha &-\sin \alpha \\\sin \alpha &\cos \alpha \end{array}}\right)\left({\begin{array}{rr}\cos \beta &-\sin \beta \\\sin \beta &\cos \beta \end{array}}\right)\\[12pt]&=\left({\begin{array}{rr}\cos \alpha \cos \beta -\sin \alpha \sin \beta &-\cos \alpha \sin \beta -\sin \alpha \cos \beta \\\sin \alpha \cos \beta +\cos \alpha \sin \beta &-\sin \alpha \sin \beta +\cos \alpha \cos \beta \end{array}}\right)\\[12pt]&=\left({\begin{array}{rr}\cos(\alpha +\beta )&-\sin(\alpha +\beta )\\\sin(\alpha +\beta )&\cos(\alpha +\beta )\end{array}}\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbbc83690fbaa9724724144f29919d3ad4f9d31c)

















![{\displaystyle {\begin{aligned}e_{0}&=1\\[6pt]e_{1}&=\sum _{i}x_{i}&&=\sum _{i}\tan \theta _{i}\\[6pt]e_{2}&=\sum _{i<j}x_{i}x_{j}&&=\sum _{i<j}\tan \theta _{i}\tan \theta _{j}\\[6pt]e_{3}&=\sum _{i<j<k}x_{i}x_{j}x_{k}&&=\sum _{i<j<k}\tan \theta _{i}\tan \theta _{j}\tan \theta _{k}\\&{}\ \ \vdots &&{}\ \ \vdots \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9fe5c7c43e0176206f134067e005328cb0a31cce)

![{\displaystyle {\begin{aligned}\tan(\theta _{1}+\theta _{2})&={\frac {e_{1}}{e_{0}-e_{2}}}={\frac {x_{1}+x_{2}}{1\ -\ x_{1}x_{2}}}={\frac {\tan \theta _{1}+\tan \theta _{2}}{1\ -\ \tan \theta _{1}\tan \theta _{2}}},\\[8pt]\tan(\theta _{1}+\theta _{2}+\theta _{3})&={\frac {e_{1}-e_{3}}{e_{0}-e_{2}}}={\frac {(x_{1}+x_{2}+x_{3})\ -\ (x_{1}x_{2}x_{3})}{1\ -\ (x_{1}x_{2}+x_{1}x_{3}+x_{2}x_{3})}},\\[8pt]\tan(\theta _{1}+\theta _{2}+\theta _{3}+\theta _{4})&={\frac {e_{1}-e_{3}}{e_{0}-e_{2}+e_{4}}}\\[8pt]&={\frac {(x_{1}+x_{2}+x_{3}+x_{4})\ -\ (x_{1}x_{2}x_{3}+x_{1}x_{2}x_{4}+x_{1}x_{3}x_{4}+x_{2}x_{3}x_{4})}{1\ -\ (x_{1}x_{2}+x_{1}x_{3}+x_{1}x_{4}+x_{2}x_{3}+x_{2}x_{4}+x_{3}x_{4})\ +\ (x_{1}x_{2}x_{3}x_{4})}},\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4c9196b8ab5e0aef6cf7d7fec7d7546f2a6e7d2f)
![{\displaystyle {\begin{aligned}\sec \left(\sum _{i}\theta _{i}\right)&={\frac {\prod _{i}\sec \theta _{i}}{e_{0}-e_{2}+e_{4}-\cdots }}\\[8pt]\csc \left(\sum _{i}\theta _{i}\right)&={\frac {\prod _{i}\sec \theta _{i}}{e_{1}-e_{3}+e_{5}-\cdots }}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f7d12744003bcf5becf301096c3d12ca1d1e1055)


![{\displaystyle {\begin{aligned}\sec(\alpha +\beta +\gamma )&={\frac {\sec \alpha \sec \beta \sec \gamma }{1-\tan \alpha \tan \beta -\tan \alpha \tan \gamma -\tan \beta \tan \gamma }}\\[8pt]\csc(\alpha +\beta +\gamma )&={\frac {\sec \alpha \sec \beta \sec \gamma }{\tan \alpha +\tan \beta +\tan \gamma -\tan \alpha \tan \beta \tan \gamma }}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e082e69aab7a9c782f590f3a93262ba3101899b7)














![{\displaystyle {\begin{aligned}\sin {\frac {\theta }{2}}&=\operatorname {sgn} \left(2\pi -\theta +4\pi \left\lfloor {\frac {\theta }{4\pi }}\right\rfloor \right){\sqrt {\frac {1-\cos \theta }{2}}}\\[3pt]\sin ^{2}{\frac {\theta }{2}}&={\frac {1-\cos \theta }{2}}\\[3pt]\cos {\frac {\theta }{2}}&=\operatorname {sgn} \left(\pi +\theta +4\pi \left\lfloor {\frac {\pi -\theta }{4\pi }}\right\rfloor \right){\sqrt {\frac {1+\cos \theta }{2}}}\\[3pt]\cos ^{2}{\frac {\theta }{2}}&={\frac {1+\cos \theta }{2}}\\[3pt]\tan {\frac {\theta }{2}}&=\csc \theta -\cot \theta =\pm \,{\sqrt {\frac {1-\cos \theta }{1+\cos \theta }}}={\frac {\sin \theta }{1+\cos \theta }}\\[3pt]&={\frac {1-\cos \theta }{\sin \theta }}={\frac {-1\pm {\sqrt {1+\tan ^{2}\theta }}}{\tan \theta }}={\frac {\tan \theta }{1+\sec {\theta }}}\\[3pt]\cot {\frac {\theta }{2}}&=\csc \theta +\cot \theta =\pm \,{\sqrt {\frac {1+\cos \theta }{1-\cos \theta }}}={\frac {\sin \theta }{1-\cos \theta }}={\frac {1+\cos \theta }{\sin \theta }}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b63b9e55447d66957c84340adb47c487c279c4ca)
![{\displaystyle {\begin{aligned}\tan {\frac {\eta \pm \theta }{2}}&={\frac {\sin \eta \pm \sin \theta }{\cos \eta +\cos \theta }}\\[3pt]\tan \left({\frac {\theta }{2}}+{\frac {\pi }{4}}\right)&=\sec \theta +\tan \theta \\[3pt]{\sqrt {\frac {1-\sin \theta }{1+\sin \theta }}}&={\frac {\left|1-\tan {\frac {\theta }{2}}\right|}{\left|1+\tan {\frac {\theta }{2}}\right|}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/639270245e163f1afa8682ac8aa12086db43dce1)







![{\displaystyle {\begin{aligned}\tan {\frac {\theta }{2}}&=\csc \theta -\cot \theta \\&=\pm \,{\sqrt {\frac {1-\cos \theta }{1+\cos \theta }}}\\[3pt]&={\frac {\sin \theta }{1+\cos \theta }}\\[3pt]&={\frac {1-\cos \theta }{\sin \theta }}\\[5pt]\tan {\frac {\eta +\theta }{2}}&={\frac {\sin \eta +\sin \theta }{\cos \eta +\cos \theta }}\\[5pt]\tan \left({\frac {\theta }{2}}+{\frac {\pi }{4}}\right)&=\sec \theta +\tan \theta \\[5pt]{\sqrt {\frac {1-\sin \theta }{1+\sin \theta }}}&={\frac {\left|1-\tan {\frac {\theta }{2}}\right|}{\left|1+\tan {\frac {\theta }{2}}\right|}}\\[5pt]\tan {\frac {\theta }{2}}&={\frac {\tan \theta }{1+{\sqrt {1+\tan ^{2}\theta }}}}\\&{\text{for }}\theta \in \left(-{\tfrac {\pi }{2}},{\tfrac {\pi }{2}}\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3cab50a58d64b8c3341fb4f7646888bbbc67ea0c)
![{\displaystyle {\begin{aligned}\cot {\frac {\theta }{2}}&=\csc \theta +\cot \theta \\&=\pm \,{\sqrt {\frac {1+\cos \theta }{1-\cos \theta }}}\\[3pt]&={\frac {\sin \theta }{1-\cos \theta }}\\[4pt]&={\frac {1+\cos \theta }{\sin \theta }}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5c9be831a310f112ffc6e5790bc705dc7b070b87)





























![{\begin{aligned}\prod _{k=1}^{n}\cos \theta _{k}&={\frac {1}{2^{n}}}\sum _{e\in S}\cos(e_{1}\theta _{1}+\cdots +e_{n}\theta _{n})\\[6pt]&{\text{where }}S=\{1,-1\}^{n}\end{aligned}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a86e836e8111fd80b7c225d1c4394bf4ac8459e5)

































![{\displaystyle {\begin{aligned}\sum _{n=1}^{N}\sin(n\theta )&={\frac {1}{2}}\cot {\frac {\theta }{2}}-{\frac {\cos \left(\left(N+{\frac {1}{2}}\right)\theta \right)}{2\sin \left({\frac {\theta }{2}}\right)}}\\[5pt]\sum _{n=1}^{N}\cos(n\theta )&=-{\frac {1}{2}}+{\frac {\sin \left(\left(N+{\frac {1}{2}}\right)\theta \right)}{2\sin \left({\frac {\theta }{2}}\right)}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/db6f6c985a9f5be2208b7618a99495233321fa02)



![{\displaystyle {\begin{aligned}\sin \varphi +\sin(\varphi +\alpha )+\sin(\varphi +2\alpha )&+\cdots \\[8pt]\cdots +\sin(\varphi +n\alpha )&={\frac {\sin {\frac {n+1}{2}}\alpha \cdot \sin \left(\varphi +{\frac {n}{2}}\alpha \right)}{\sin {\frac {1}{2}}\alpha }}\quad {\text{and}}\\[10pt]\cos \varphi +\cos(\varphi +\alpha )+\cos(\varphi +2\alpha )&+\cdots \\[8pt]\cdots +\cos(\varphi +n\alpha )&={\frac {\sin {\frac {n+1}{2}}\alpha \cdot \cos \left(\varphi +{\frac {n}{2}}\alpha \right)}{\sin {\frac {1}{2}}\alpha }}.\\[10pt]\sec x\pm \tan x&=\tan \left({\frac {\pi }{4}}\pm {\frac {1}{2}}x\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d2de90e95fd75c1b8e9e596a63d89e6f651ad7f3)



































![{\displaystyle {\begin{aligned}\cos {\frac {2\pi }{21}}+{}&\cos \left(2\cdot {\frac {2\pi }{21}}\right)+\cos \left(4\cdot {\frac {2\pi }{21}}\right)\\[10pt]{}+{}&\cos \left(5\cdot {\frac {2\pi }{21}}\right)+\cos \left(8\cdot {\frac {2\pi }{21}}\right)+\cos \left(10\cdot {\frac {2\pi }{21}}\right)={\frac {1}{2}}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1a273cb1f3cc75f7f1297ddd3e91b361d8c0854b)
















![{\displaystyle {\begin{matrix}\sin \left(0\right)&=&\sin \left(0^{\circ }\right)&=&{\dfrac {\sqrt {0}}{2}}&=&\cos \left(90^{\circ }\right)&=&\cos \left({\dfrac {\pi }{2}}\right)\\[5pt]\sin \left({\dfrac {\pi }{6}}\right)&=&\sin \left(30^{\circ }\right)&=&{\dfrac {\sqrt {1}}{2}}&=&\cos \left(60^{\circ }\right)&=&\cos \left({\dfrac {\pi }{3}}\right)\\[5pt]\sin \left({\dfrac {\pi }{4}}\right)&=&\sin \left(45^{\circ }\right)&=&{\dfrac {\sqrt {2}}{2}}&=&\cos \left(45^{\circ }\right)&=&\cos \left({\dfrac {\pi }{4}}\right)\\[5pt]\sin \left({\dfrac {\pi }{3}}\right)&=&\sin \left(60^{\circ }\right)&=&{\dfrac {\sqrt {3}}{2}}&=&\cos \left(30^{\circ }\right)&=&\cos \left({\dfrac {\pi }{6}}\right)\\[5pt]\sin \left({\dfrac {\pi }{2}}\right)&=&\sin \left(90^{\circ }\right)&=&{\dfrac {\sqrt {4}}{2}}&=&\cos \left(0^{\circ }\right)&=&\cos \left(0\right)\\[5pt]&&&&\uparrow \\&&&&{\text{These}}\\&&&&{\text{radicands}}\\&&&&{\text{are}}\\&&&&0,\,1,\,2,\,3,\,4.\end{matrix}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0abb840d75498e4216fb9d4237ba1de5b64421a1)
![{\displaystyle {\begin{aligned}\cos {\frac {\pi }{5}}&=\cos 36^{\circ }={\frac {{\sqrt {5}}+1}{4}}={\frac {\varphi }{2}}\\[3pt]\sin {\frac {\pi }{10}}&=\sin 18^{\circ }={\frac {{\sqrt {5}}-1}{4}}={\frac {\varphi ^{-1}}{2}}={\frac {1}{2\varphi }}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/779dfef7b48f2ae8cc0d676651c534b3b78c7384)









![{\displaystyle {\begin{aligned}\int {\frac {du}{\sqrt {a^{2}-u^{2}}}}&=\sin ^{-1}\left({\frac {u}{a}}\right)+C\\[3pt]\int {\frac {du}{a^{2}+u^{2}}}&={\frac {1}{a}}\tan ^{-1}\left({\frac {u}{a}}\right)+C\\[3pt]\int {\frac {du}{u{\sqrt {u^{2}-a^{2}}}}}&={\frac {1}{a}}\sec ^{-1}\left|{\frac {u}{a}}\right|+C\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ef0d9112c732201db64cd2b2c290548a2611b791)
























