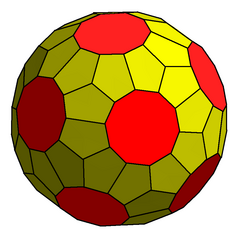
Truncated triakis icosahedron
| Truncated triakis icosahedron | |
|---|---|
| |
| Conway notation | t10kI = dk10tD |
| Faces | 12 decagons 60 pentagons |
| Edges | 210 |
| Vertices | 140 |
| Dual | Decakis truncated dodecahedron |
| Vertex configuration | 12 (5.5.5) 60 (5.5.10) |
| Symmetry group | Ih |
| Properties | convex |
 Net | |
The truncated triakis icosahedron, or more precisely an order-10 truncated triakis icosahedron, is a convex polyhedron with 72 faces: 10 sets of 3 pentagons arranged in an icosahedral arrangement, with 12 decagons in the gaps.
Triakis icosahedron[]
It is constructed from taking a triakis icosahedron by truncating the order-10 vertices. This creates 12 regular decagon faces, and leaves 60 mirror-symmetric pentagons.
 Triakis icosahedron |
Decakis truncated dodecahedron[]
The dual of the truncated triakis icosahedron is called a decakis truncated dodecahedron. It can be seen as a truncated dodecahedron with decagonal pyramids augmented to the faces.
 Truncated dodecahedron |
 Decakis truncated dodecahedron |
 Net |
See also[]
External links[]
 | This polyhedron-related article is a stub. You can help Wikipedia by . |
Categories:
- Polyhedra
- Truncated tilings
- Polyhedron stubs